Question

Question #5b9c1

Answer 1

I think you have a small misunderstanding the force you have given is not non-conservative at all, in fact its a conservative force.
Your question should have actually been " Why is the force `1 hat i - 1 hat j` conservative?"

The most general way to prove that any given force is conservative is to consider two points and to find out the work done by the given force along any arbitrary curve joining these two points. If the work only depends on the coordinates of the end points, the force given is conservative else, if it depends on the curve along which work was calculated its non conservative.

The tricky part is, to prove that a force is conservative you need find out the work along all possible paths connecting the two points, and that means finding out the work done along infinite number of curves. But a little mathematical cleverness helps us get around it.

Note: I am really sorry, I couldn't find a few mathematical symbols hence I uploaded the ones written by me. If any one could convert them into mathematical symbols it would be a huge help.

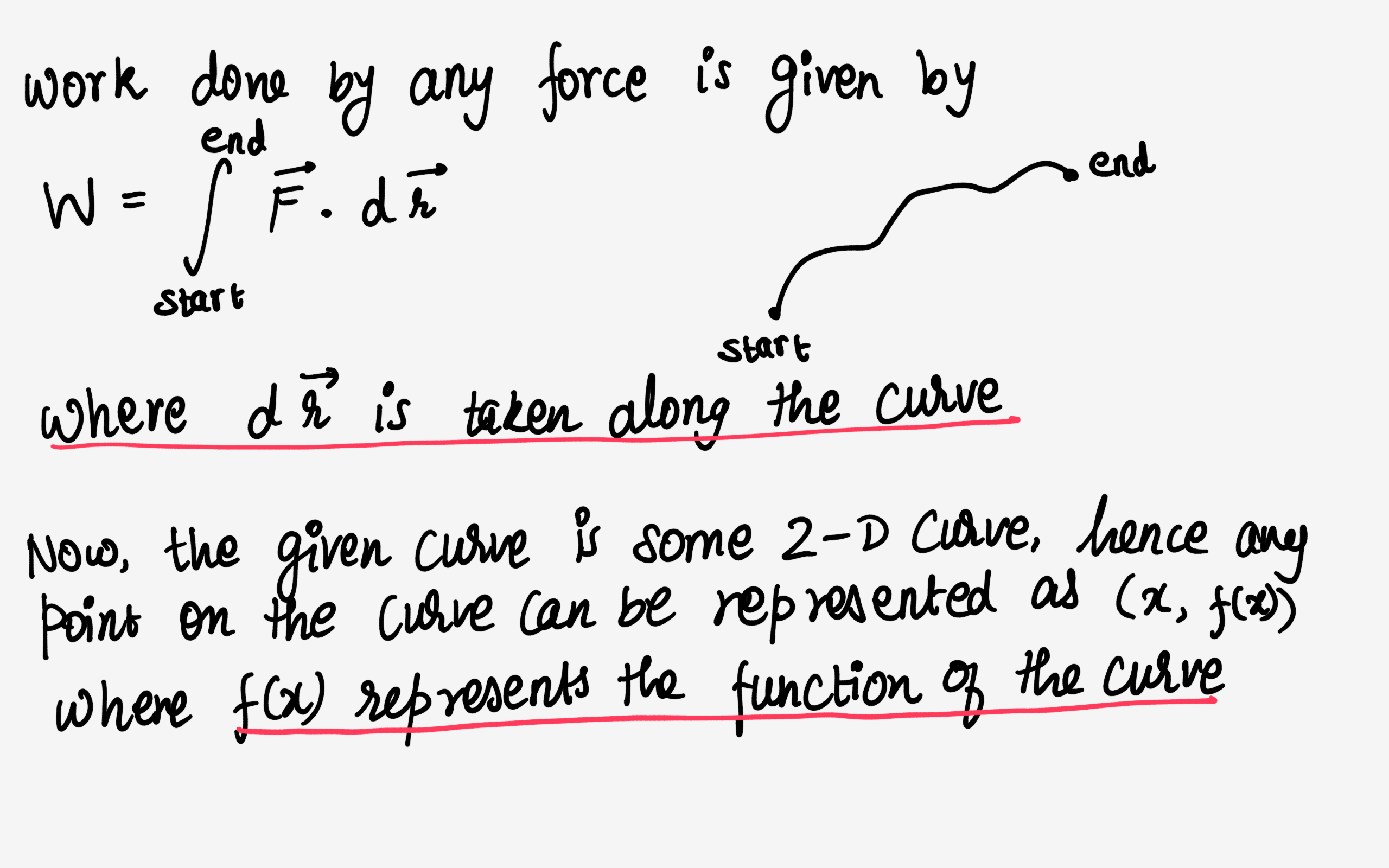
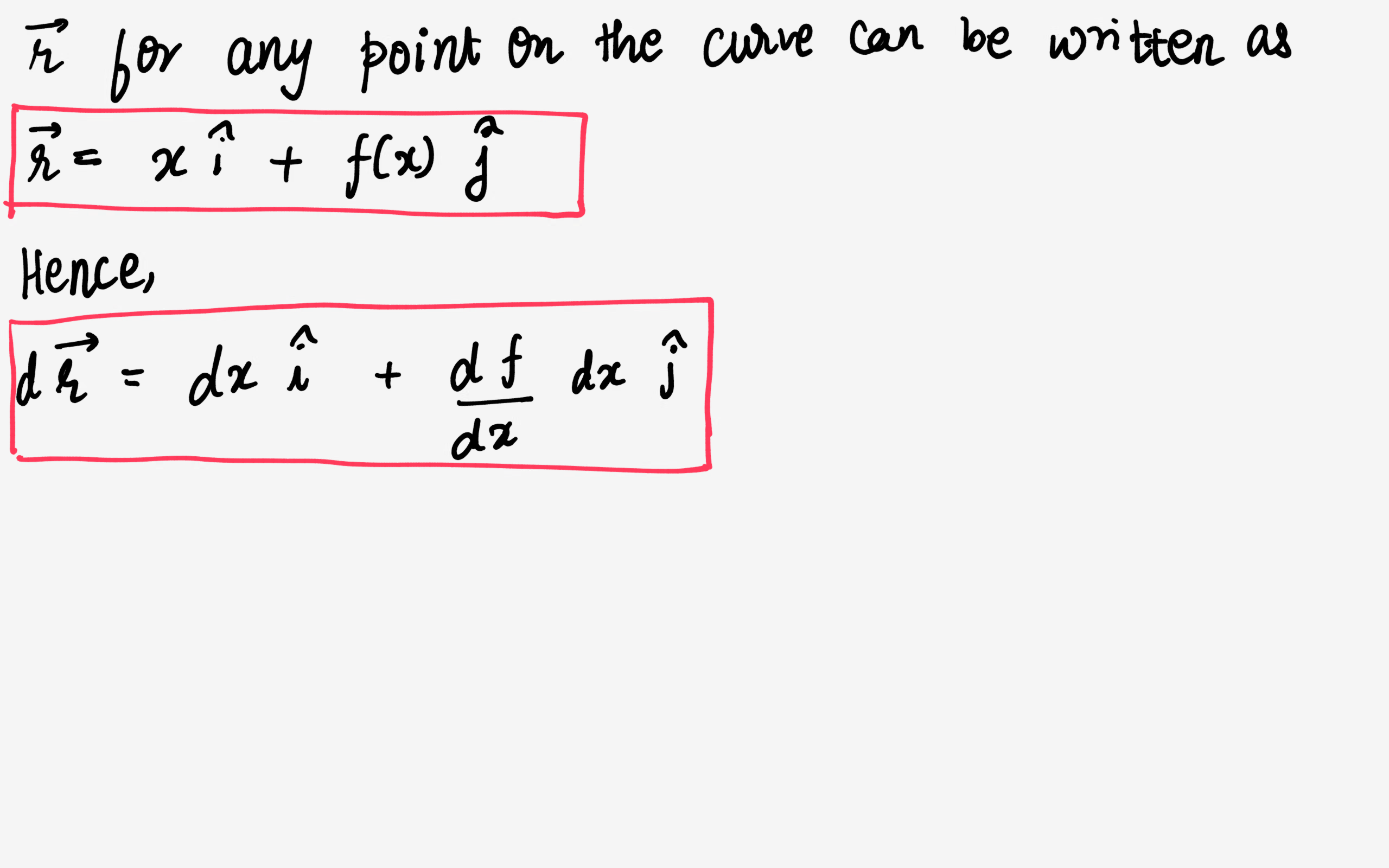
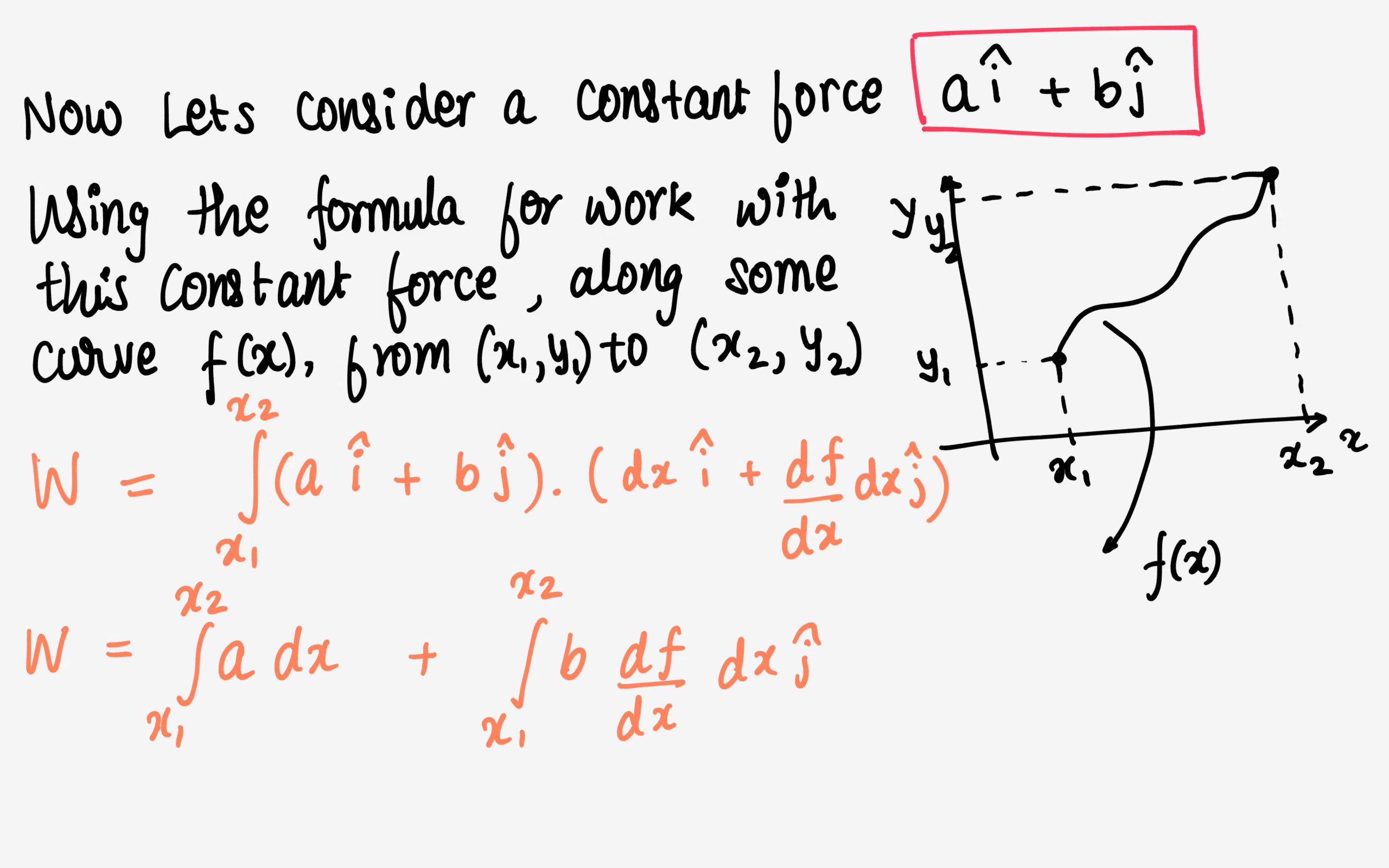
And the above result should not surprising at all, after all constant forces like the gravity on the surface of the earth (i.e. the force on a object near the surface of the earth is mass `xx` g which is a constant force)is after all a conservative force.

Hence we have comprehensively proved "any constant force is a conservative force".

There is much more general method that physicists would actually prefer and that would be the existence of potential. If we successfully find a potential whose gradient is the given force then we would have successfully proven that the given force is Conservative. But this method is more mathematically involved and involves the use of partial derivatives and gradients etc. I would motivate all of those who have understood this method to take a look at that method as well.