How do you find the exact value of the trigonometric function cos 570 degrees?
2 Answers
There exists 4 trigonometric quadrants. All, Sine, Tan & Cos .
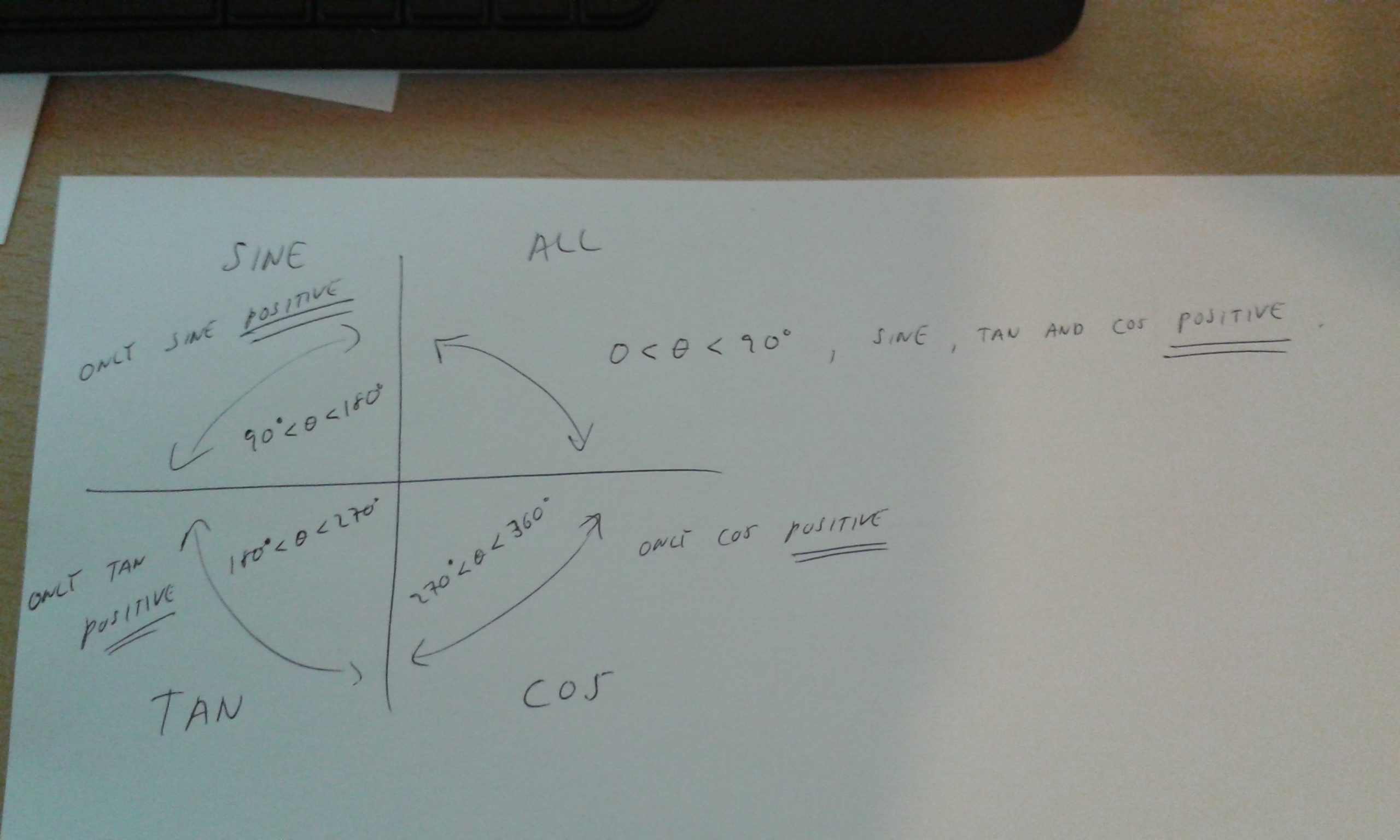
570 degrees turns out to land in the tan quadrant, therefore cos(570) has to be negative.
90 (all) + 90 (sin) + 90 (tan) + 90 (cos) + 90 (all) + 90 (sin) + 30 (tan) makes 570 degrees and the last turn helps us land in the tan quadrant.
Remember that 90+90+90+90+90+90+30=570.
So we have to create a 30 degree right angled triangle in the tan quadrant.
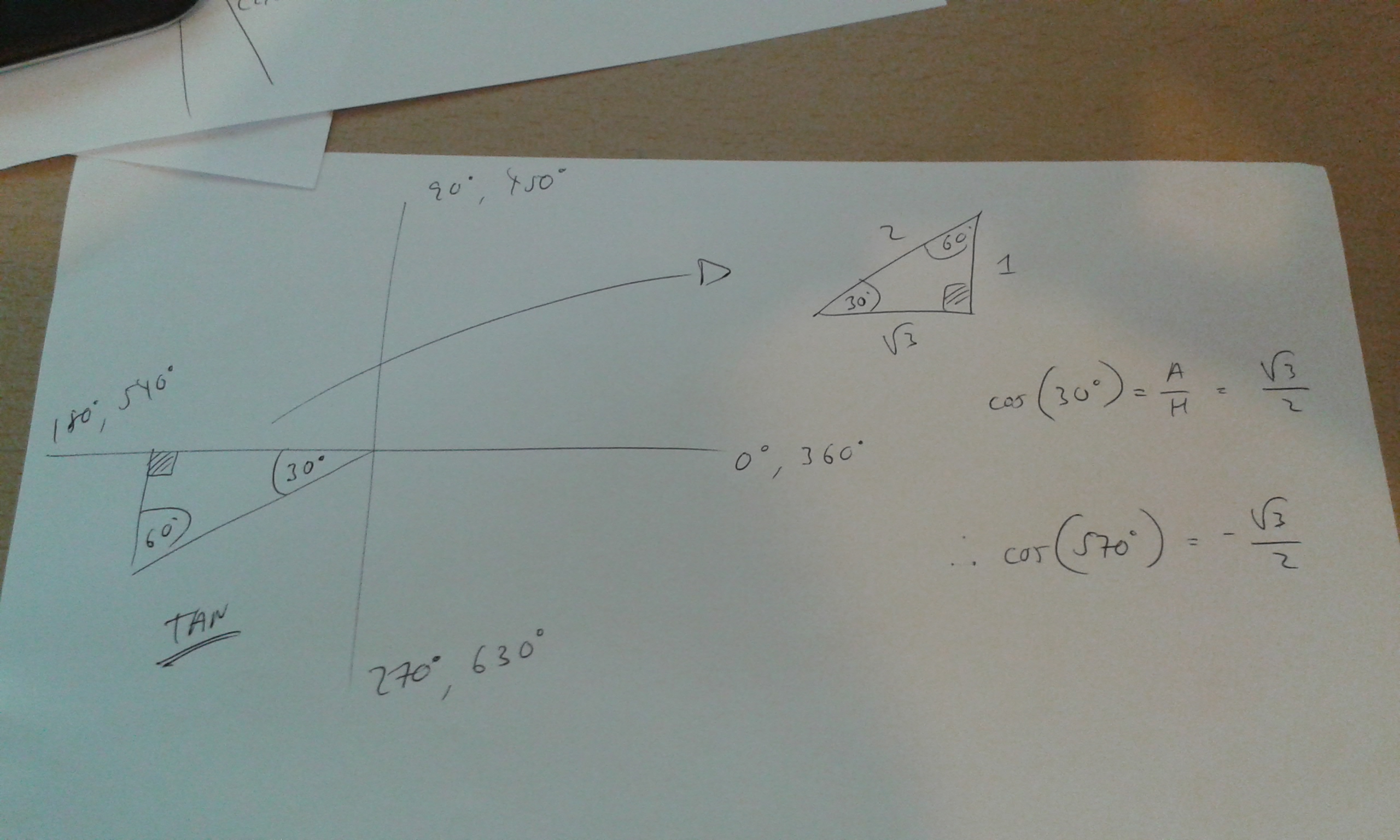
We then figure out the value of the adjacent side to this triangle and its hypotenuse to get the value of cos(570) which is equal to
Use the trig unit circle as proof.
=
or...


