Question

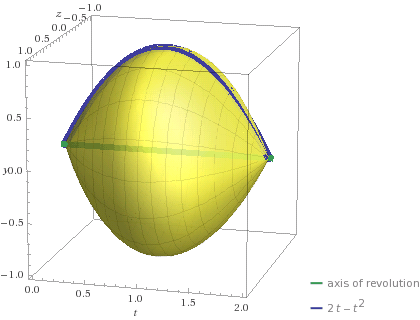
The area bounded by the curve `y=3+2x-x^2` and line `y=3` is rotated completely about the line `y=3`. Find the volume of the solid of revolution obtained?

Answer 1

`V=16/15pi~~3.35103`

Explanation:

The area are the solution of this system:
`{(y<=-x^2+2x+3),(y>=3):}`
And it is sketched in this plot:

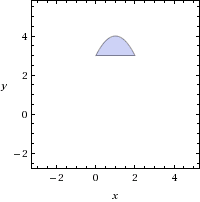
The formula for the volume of a x-axis rotation solid is:
`V=pi*int_a^b f^2(z) dz`.

To apply the formula we should translate the half moon on the x-axis, the area won't change, and so it won't change also the volume:
`y=-x^2+2x+3color(red)(-3)=-x^2+2x`
`y=3color(red)(-3)=0`
In this way we obtain `f(z)=-z^2+2z`.
The translated area now is plotted here:

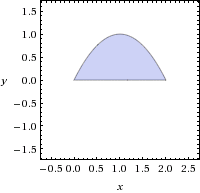
But which are the a and b of the integral? The solutions of the system:
`{(y=-x^2+2x),(y=0):}`
So `a=0 and b=2`.

Let's rewrite and solve the integral:
`V=pi*int_0^2 (-z^2+2z)^2 dz`
`V=pi*int_0^2 z^4-4z^3+4z^2 dz`
`V=pi*[z^5/5-(4z^4)/4+(4z^3)/3]_0^2`
`V=pi*[z^5/5-z^4+(4z^3)/3]_0^2`
`V=pi*(2^5/5-2^4+(4*2^3)/3-0^5/5+0^4-(4*0^3)/3)`
`V=pi*(32/5-16+32/3+0)`
`V=pi*(96/15-240/15+160/15)`
`V=pi*(96/15-240/15+160/15)`
`V=16/15pi~~3.35103`
And this "lemon" is the solid obtained: