How do you solve the series #sin (1/n)# using comparison test?
1 Answer
Jul 2, 2015
By comparing it with
Explanation:
The sine function has this weird property that for very small values of
You can see this easily by plotting the graph for
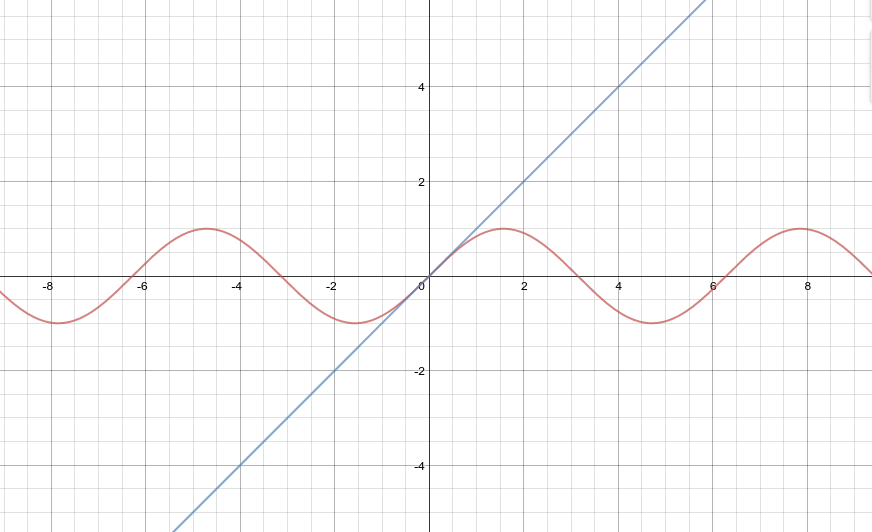
You can see that when
So this also means that for very small values of
When does
We also know that

