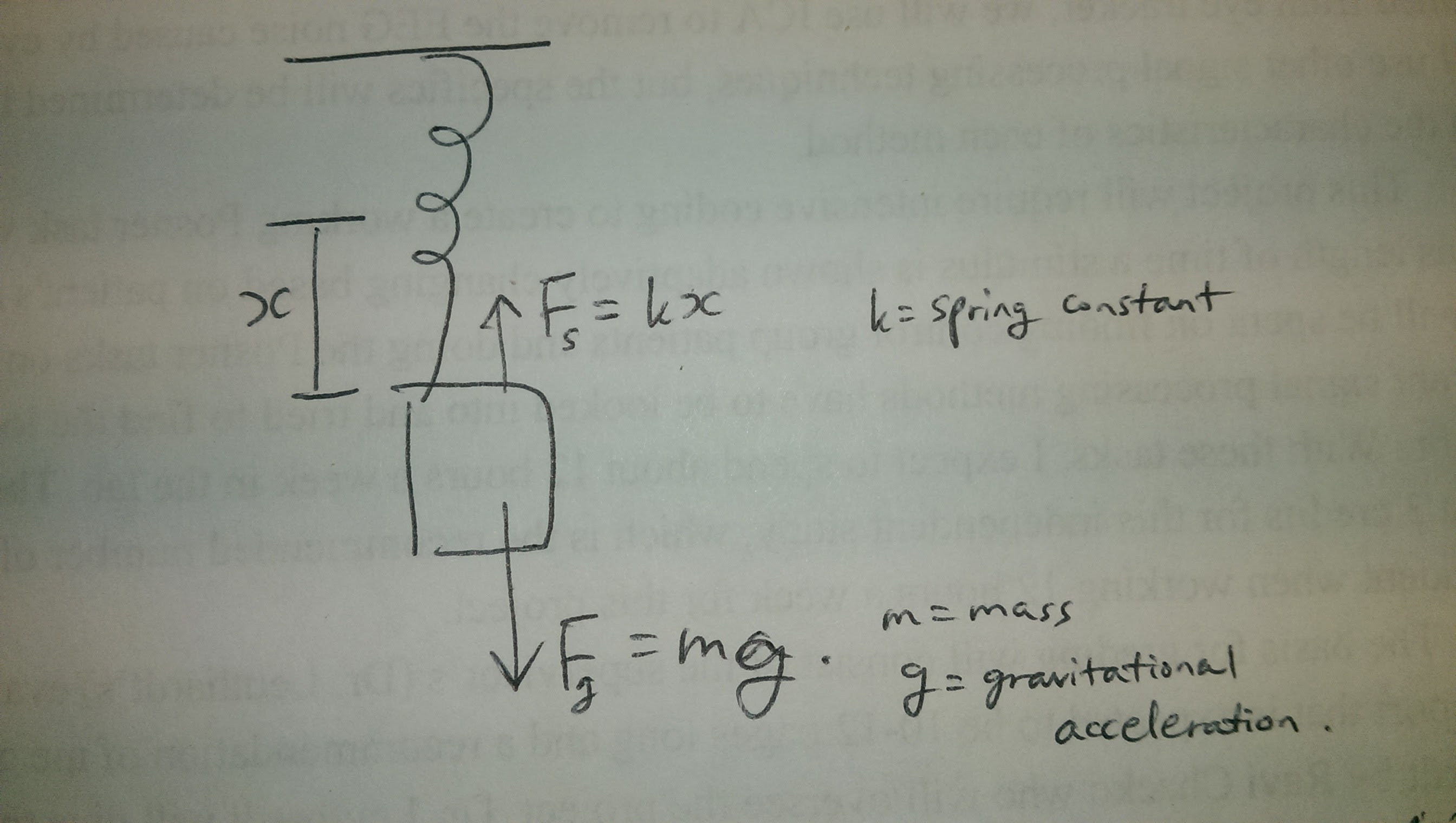
At any time, the net force is gravitational force - spring force.
Thus, I write this equation: #m(d^2x)/dt^2+10x=mg#
10 is the spring constant k.
The equation for this system is
#x(t)=c_4cos(sqrt(10/m)t)+c_5sin(sqrt(10/m)t)+mg#.
(If you want to know more about how this is derived. Look below to Heavy Math Part section.)
#c_4# and #c_5# cannot be determined without more information, such as value of #x(0)# when #t=0#.
However, we can still use this equation to find the oscillation frequency. The only oscillatory components are #c_4cos(sqrt(10/m)t)# and #c_5sin(sqrt(10/m)t)#.
For frequency to be #1#, #sqrt(10/m)=2pi# which means #m=0.253#.
Since frequency is not dependent on #g#, the mass to create frequency of #1s^-1# or period of #1s# would be #0.253 kg# on both Earth and Mars.
Heavy Math Part
So what we have now is a second-order nonhomogeneous differential equation.
We first solve the left-hand side. That is: #m(d^2x)/dt^2+10x=0#
Substituting #dx/dt# with #s# gives #ms^2+10=0#.
Solving for #s# gives #s=sqrt(10/m)i, -sqrt(10/m)i#.
The general solution becomes #x(t)=c_1e^(sqrt(10/m)i)+c_2e^(-sqrt(10/m)i)+c_3#.
Substituting Euler's formula #e^(it)=cos(t)+isin(t)# gives #x(t)=c_1(cos(sqrt(10/m)t)+isin(sqrt(10/m)t))+c_2(cos(sqrt(10/m)t)-isin(sqrt(10/m)t))+c_3#.
To get rid of imaginary components, we assume #c_1# and #c_2# to be multiples of certain #c_1# and #c_2# values.
If #c_1=c_2=c_4/2#, #x(t)=c_4cos(sqrt(10/m)t)#.
If #c_1=c_5/(2i)# and #c_2=-c_5/(2i)#, #x(t)=c_5sin(sqrt(10/m)t)#
Now we have an equation without imaginary components:
#x(t)=c_4cos(sqrt(10/m)t)+c_5sin(sqrt(10/m)t)+c_3#.
We are done with the left side of the first equation. Now we find #c_3# using right side of the first equation.
#(d^2x)/dt^2=-10c_4/mcos(sqrt(10/m)t)-10c_5/msin(sqrt(10/m)t)#
#m(d^2x)/dt^2+10x=-10c_4cos(sqrt(10/m)t)-10c_5sin(sqrt(10/m)t)+10c_4cos(sqrt(10/m)t)+10c_5sin(sqrt(10/m)t)+c_3=c_3=mg#
Thus, #x(t)=c_4cos(sqrt(10/m)t)+c_5sin(sqrt(10/m)t)+mg#.

