What is the area of an equilateral triangle with height of 9 inches?
1 Answer
Explanation:
In such situations, the first step is to draw a picture.
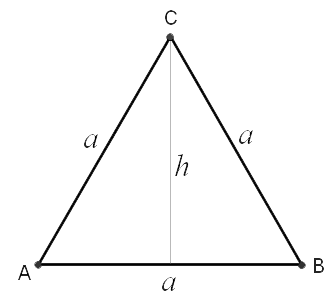
In relation to the notation introduced by the picture, we know that
Knowing that the triangle is equilateral makes everything easier: the heights are also medians. So the height
Then, the triangle is divided into two congruent right triangles and the Pythagorean Theorem holds for one of these two right triangles:
Now the area:

