How do you graph #4x^2+4y^2-16x-16y+23=0 #?
1 Answer
Center:
Explanation:
First, complete the square to write the equation in standard form of an ellipse
The goal in completing the square to create perfect trinomial in either one of this form
#(a^2 + 2ab+ b^2) = (a+b)^2#
#(a^2 -2ab +b^2) = (a-b)^2#
Given
Rewrite as follow:
#4(x^2 -4x) + 4(y^2 -4y) = -23#
#4(x^2 -4x + color(red)(4)) + 4(y^2 -4y + color(red)(4)) = -23 + color(red)(16 + 16)#
# 4(x-2)^2 + 4(y-2)^2 = 9#
Divide both side by 9, to get the general form of an ellipse
#(4(x-2)^2)/9 +( 4(y-2)^2)/9 = 9/9 #
#(x-2)^2 /(9/4) +(y-2)^2/(9/4) = 1#
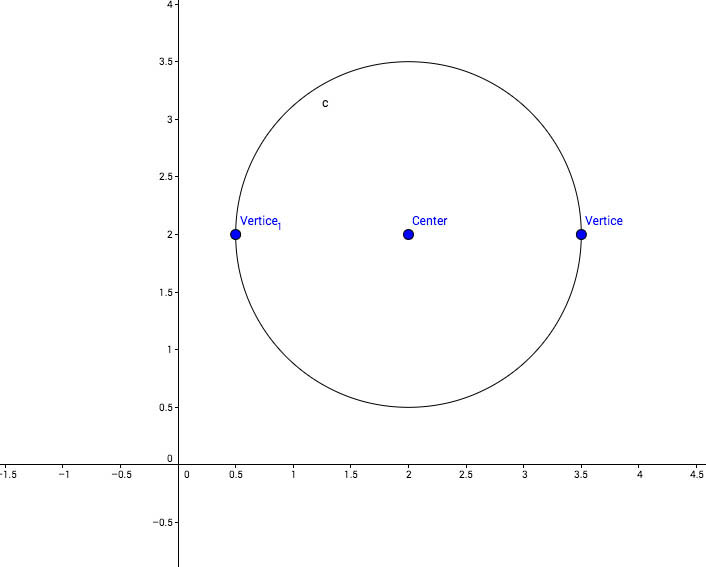
Center:
#b^2 = 9/4 = b = 3/2#
# c^2 = a^2 - b^2 = 9/4 -9/4 #
#c= 0#
Foci:
# (2-0, 0) => (2,2)#
Vertices:
#(2 + 3/2 , 2) => (7/2 , 2)#
To graph, plot the center first.
Then from the center to left 1.5, right 1.5
Then from center go up 1.5 and down 1.5
Then connect those point as best as you can
This ellipse look like a circle because eccentricity is