Question

How do you integrate `int (4^(7x)) / (5^(2x))` using integration by parts?

Answer 1

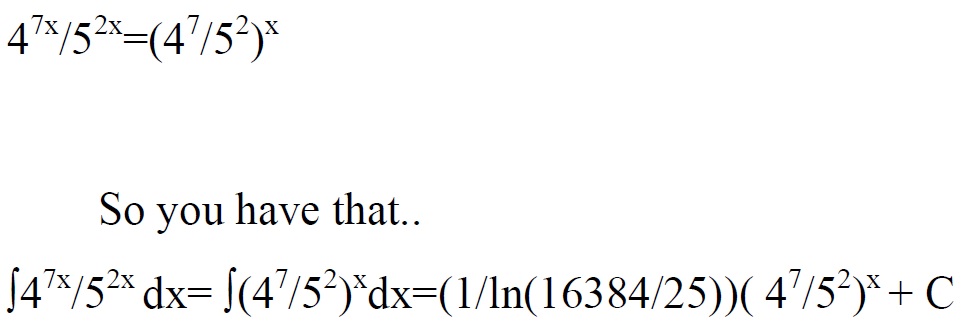
Explanation:

Hi!
I attached a quick jpg image of what you should do.

I'll get more familiar with Socratic's proprietary math editor, but hopefully this will suffice for now. Just use properties of exponents and integrate. You can use parts, but I believe that is much more involved and sort of counterproductive.

Let me know if this makes sense!

Ken

Answer 2

`int frac{4^{7x}}{5^{2x}} dx = frac{frac{4^{7x}}{5^{2x}}}{14ln2-2ln5} + C`

where `C` is a constant of integration

Explanation:

`int frac{4^{7x}}{5^{2x}} dx = int (frac{4^7}{5^2})^x dx`

`= int e^{ln(frac{4^7}{5^2})x} dx`

`= int e^{(14ln2-2ln5)x} dx`

`= frac{e^{(14ln2-2ln5) x}}{14ln2-2ln5} + C`

where `C` is a constant of integration

`= frac{frac{4^{7x}}{5^{2x}}}{14ln2-2ln5} + C`

Answer 3

`I =(4^(7x)*5^(-2x))/(7ln(4)+2ln(5))+c`

Explanation:

Since you did ask for integration by parts

`I = int4^(7x)/5^(2x)dx`

Or, rewriting it a little

`I = inte^(7*ln(4)*x)*e^(-2*ln(5)*x)dx`

Saying `u = e^(7*ln(4)*x)` we have `du = 7ln(4)*e^(7*ln(4)*x)`
And `dv = e^(-2*ln(5)*x)` so `v = -e^(-2*ln(5)*x)/(2*ln(5)`

`I = e^(7*ln(4)*x-2*ln(5)*x)/(2*ln(5)) - (7ln(4))/(2ln(5))inte^(7*ln(4)*x-2*ln(5)*x)dx`

The latter integral is the same as `I` so we have

`(7ln(4)+2ln(5))/(2ln(5))I = e^(7*ln(4)*x-2*ln(5)*x)/(2*ln(5))`

`I = e^(7*ln(4)*x-2*ln(5)*x)/(7ln(4)+2ln(5))+c`
`I =(4^(7x)*5^(-2x))/(7ln(4)+2ln(5))+c`