Question

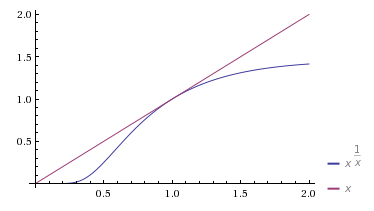
What is the equation of the line tangent to `f(x)=x^(1/x)` at `x=1`?

Answer 1

`y = x`

Explanation:

To find the tangent's slope just derivate the function.

We have
`y = x^(1/x)`

So take the log of both sides,
`ln(y) = ln(x)/x`

Derivate both sides,

`dy/dx*1/y= 1/x*d/dxln(x)+ln(x)*d/dx1/x`

`dy/dx*1/y = 1/x^2 - ln(x)*1/x^2`

`dy/dx = y*(1-ln(x))/x^2 = x^(1/x-2)*(1-ln(x))`

To find the slope at `x=1`, just switch it in, so:

`dy/dx = 1^(1/1-2)\*(1-ln(1)) = 1^(-1)*(1-0) = 1`

We know all lines follow the equation

`y = mx + b`

We know `y`, `x` and `m`, all that's left is discovering `b`

`(1)^(1/1) = 1*1 + b`
`1 = 1 + b`
`b = 0`

So the tangent line is

`y = x`