Question

Question #11ae5

Answer 1

The time period is less than as when the elevator is at rest.

Explanation:

The time period `T` of a simple pendulum, for small angle of swing, is given by the expression
`T=2 pi sqrt(l/g)`,
where `l and g` are the length of the string to which bob is attached and acceleration due to gravity respectively.

While deriving this expression it is presumed that the other end of the string is tied to a frame which is stationary and gravity is the only force acting on the bob.

Suppose the other end is tied to ceiling of an elevator which is ascending with a constant acceleration `a`. We know that acceleration will exert force on the bob, as such now it is under two forces. Therefore, now the time period `T'` will be given by

`T'=2 pi sqrt(l/(g'))`
where `g'` is the effective acceleration acting on the bob. To find out effective acceleration, let us look at the picture below

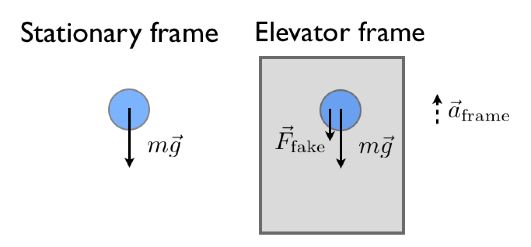
In accelerating frames, like this elevator, laws of Physics are not applicable as we know these are applicable in non-accelerating frames.

There is no direct interaction between the ascending elevator and the bob. With the upward movement of the elevator, the bob 'feels left behind'. Therefore, we need to add fake force as shown in the picture. From Newton's Second Law we know that force is directly proportional to the mass of the object.
`vecF_"Fake"=mcdot veca_"Elevator"`

Therefore, at the equilibrium state of a pendulum, when the bob is not swinging, we have the equation such that

`vecF_"Total" = vecF_"Fake"+vec F_"gravity"=mveca_"Elevator" + mvecg`
Both `veca and vecg` acting in the same direction, adding the magnitudes of both we obtain magnitude of effective force as

`:. F_"effective"= m(a+g)`

In general, the effective acceleration `g'` in an ascending elevator is

`g' = (a + g)`.

Therefore, the period of the pendulum in an ascending elevator is
`T'=2 pi sqrt(l/((a + g)))`

Since the denominator has increased, time period becomes less than when the elevator is at rest.