What are the intercepts of #-y=3y-6x-9#?

Question

1938 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-04-12T18:31:14

#y#-intercept is #(0,2.25)#

#x#-intercept is #(-1.5,0)#

The #y#-intercept is the point where the line cuts the #y#-axis.
This means, we have to find the point when #x=0#.

Similarly, the #x#-intercept is the point where the line cuts the #x#-axis. i.e. to find the point when #y=0#.

Pretty simple.

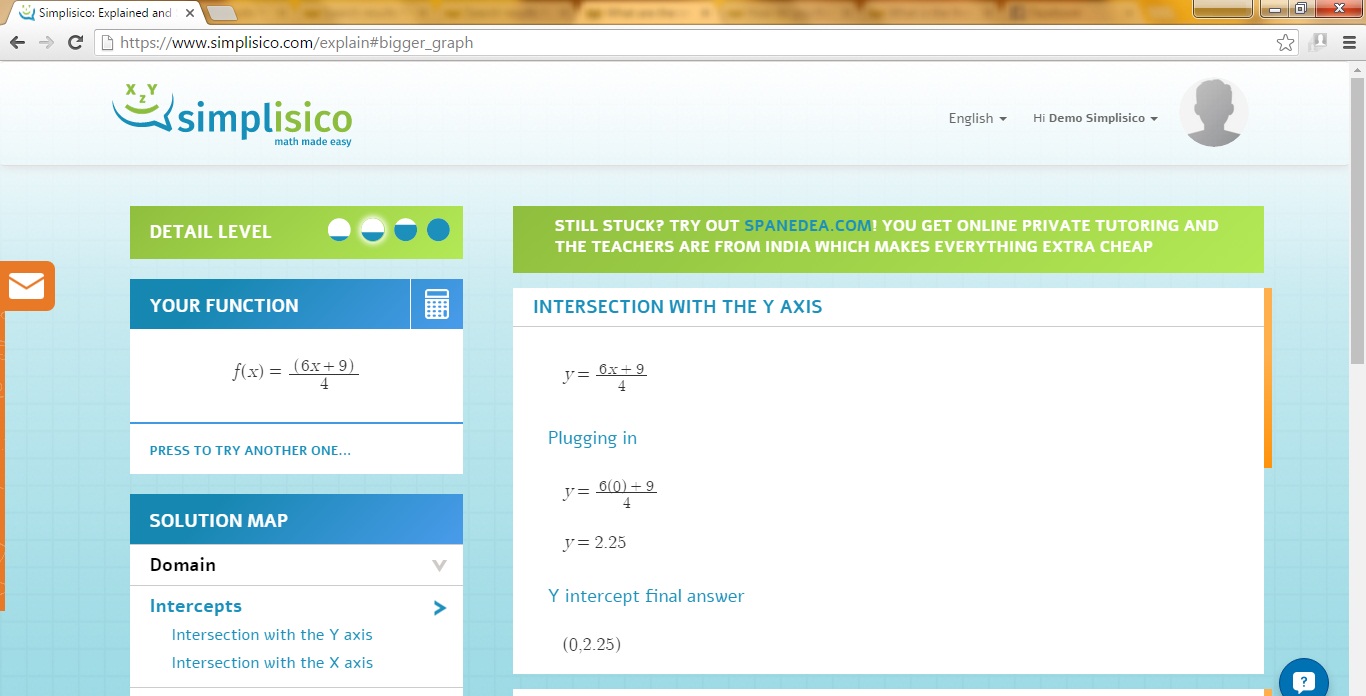
Here, first let's write the equation in terms of #y#.

#-y=3y-6x-9#

#=>-3y-y=-6x-9#

Multiply both sides by #-1#

#=>3y+y=6x+9#

#=>4y=6x+9#

#=>y=(6x+9)/4#

Below is a step-by-step to work out the #y#-intercept and #x#-intercept .

enter image source here

The graph shows the line cutting the two axes.