In a scale model of the Local Group of Galaxies, in which the Milky Way Galaxy was the size of a marble (about 1 cm in diameter), how far would it be from the Milky Way Galaxy to the most distant galaxies in the observable universe on this scale?
1 Answer
Roughly
Explanation:
We can start with taking the size of the Milky Way Galaxy and relating that size to the size of a marble and then from that calculate the distance between our marble and those of the most distant galaxies in the Universe.
The first thing to know is that, if we were being accurate about it, our marble wouldn't be round at all but much more like an air hockey puck. The Milky Way Galaxy is roughly
https://en.wikipedia.org/wiki/Milky_Way
But let's run with the conversion of:
The next question is to ask is Where are we measuring to? There are two references to distances in the question - the Local Group of Galaxies and the furthest galaxies in the observable Universe. So let's look at both and see what we get.
The Local Group is a group of galaxies in close proximity to our own and has as it's notable members the Andromeda and Triangulum galaxies. https://en.wikipedia.org/wiki/Local_Group
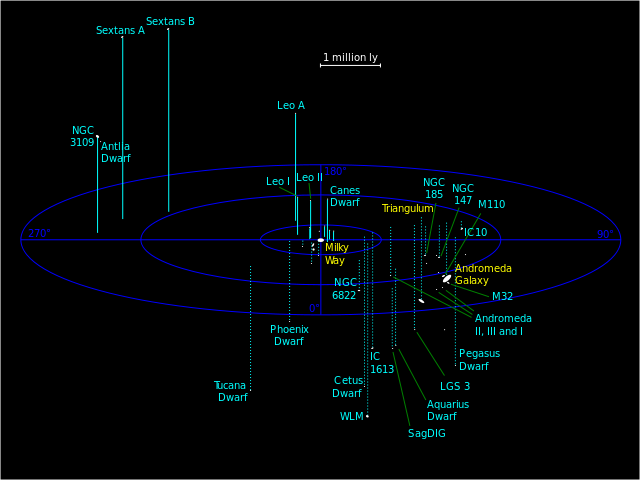
The Andromeda Galaxy is roughly
Ok - so that's a look at the Local Group. Now let's go to the edges of the observable universe .
As our observational tools improve, the distance we can see increases. The latest news from Hubble on how far it can see is from this article in March 2016 is a galaxy 13.3 BLY away. http://www.space.com/32150-farthest-galaxy-smashes-cosmic-distance-record.html
So let's see where we'd put that marble:
To put this into perspective, the distance between the two marbles would be about


