How do you find the area bounded by #y=x+4# and #y=x^2+2#?

Question

14921 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-10-31T17:09:47

Please see the explanation. #A = 4.5 units^2#

Given:
#y = x + 4# [1]
#y = x^2 + 2# [2]

We need to find the points of intersection.

Subtract equation [1] from equation [2]:

#y - y = x^2 -x + 2 - 4#

#0 = x^2 -x - 2#

#0 = (x -2)(x +1)#

#x = -1 and x = 2#

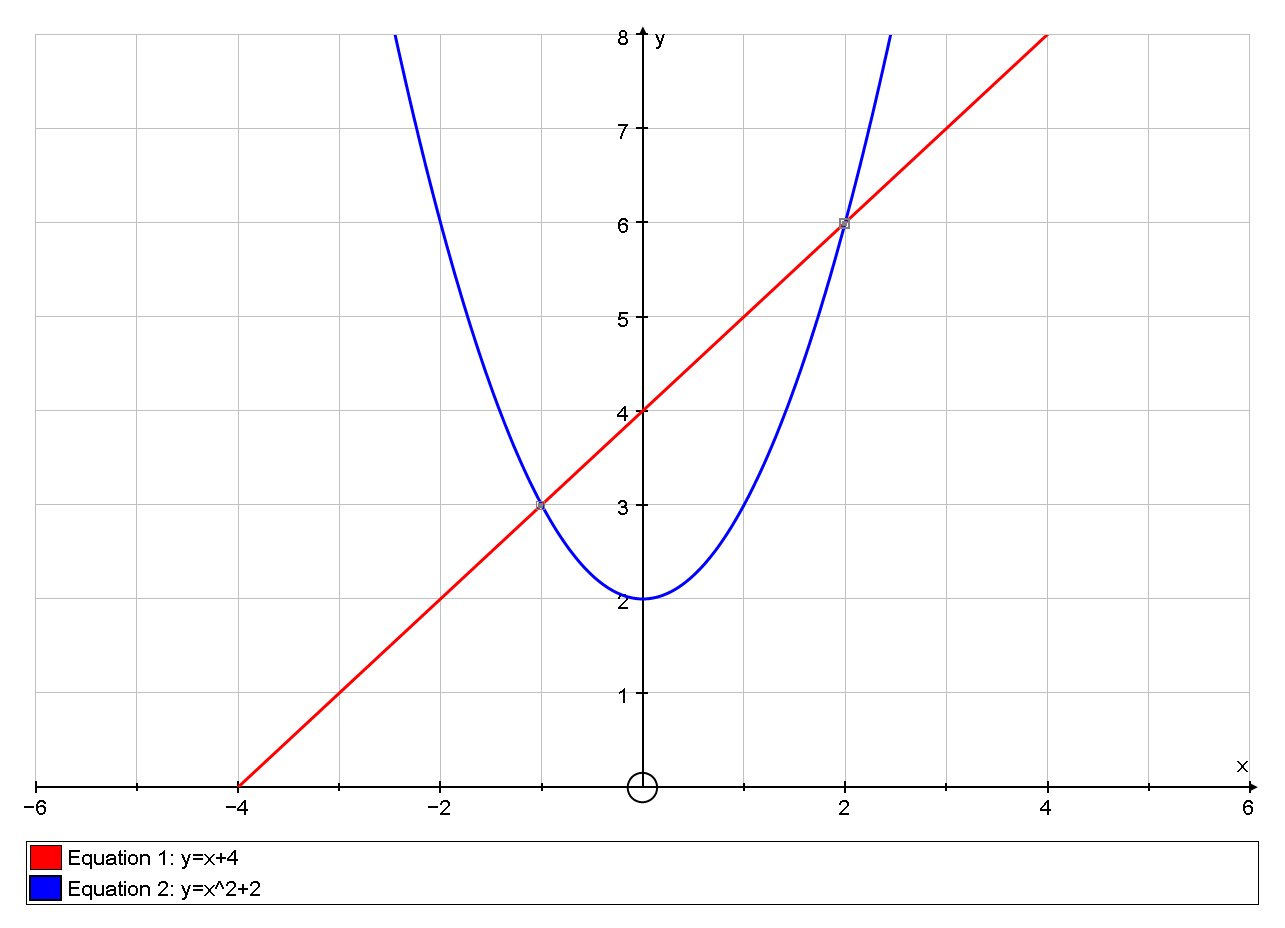
enter image source here

The line is greater than the parabola in the region #-1 < = x <= 2#, therefore, the integral for the area, A, is:

#A = int_-1^2((x + 4) - (x^2 + 2))dx#
#A = int_-1^2 (-x^2 + x + 2) dx#
#A = -x^3/3 + x^2/2 + 2x|_-1^2#
#A = 4.5 units^2#