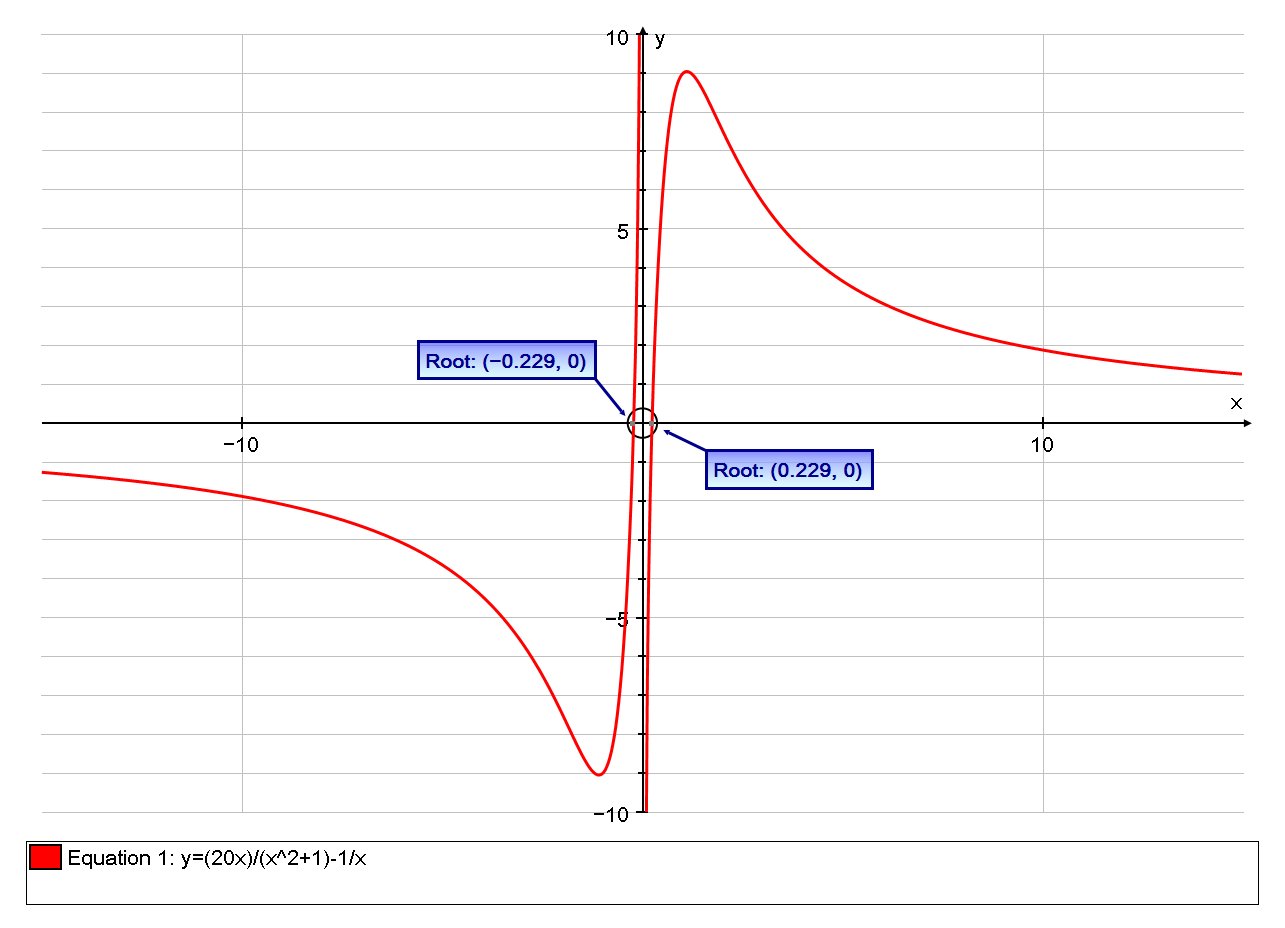
How do you find intercepts, extrema, points of inflections, asymptotes and graph #y=(20x)/(x^2+1)-1/x#?
1 Answer
There is a vertical asymptote at
There is a horizontal asymptote as
x-intercepts when
y(x) is an odd function, and it is symmetric about the origin O
Critical points are:
Maximum at
Minimum at
Explanation:
# y=(20x)/(x^2+1)-1/x #
# :. y=((20x^2)-(x^2+1))/(x(x^2+1)) #
# :. y=(19x^2-1)/(x(x^2+1)) #
Vertical Asymptotes
These will occur when the denominator is zero
# :. x(x^2+1)) =0 => x=0 #
So There is a vertical asymptote at
Horizontal Asymptotes
We need to examine the behaviour as
For large
So,
So There is a horizontal asymptote as
Odd/Even Function
Find y(-x);
# y(-x)=(19(-x)^2-1)/(-x((-x)^2+1)) #
# y(-x)=(19x^2-1)/(-x(x^2+1)) #
# y(-x)=-(19x^2-1)/(x(x^2+1)) #
# y(-x)=-y(x) #
Hence y(x) is an odd function, and it is symmetric about the origin O
Intercepts
# y=(19x^2-1)/(x(x^2+1)) => 19x^2-1 = 0 #
# :. x^2=1/19 #
# :. x^2=+-1/sqrt19 ~~~ +- 0.229#
We have already established that x=0 is a vertical asymptotes so there are not y-axis intercepts.
Hence x-intercepts when
Summary
There is a vertical asymptote at
There is a horizontal asymptote as
y(x) is an odd function, and it is symmetric about the origin O
Extrema
Because of symmetry we only need to identify extra for
We can write;
# y=(19x^2-1)/(x^3+x) #
Using the quotient rule
# y=(19x^2-1)/(x^3+x) #
# dy/dx=((x^3+x)(d/dx(19x^2-1)) - (19x^2-1)(d/dx(x^3+x)) )/(x^3+x)^2 #
# :. dy/dx=((x^3+x)(38x) - (19x^2-1)(3x^2+1) )/(x^3+x)^2 #
# :. dy/dx=(38x^4+38x^2 - (57x^4+16x^2-1) )/(x^3+x)^2 #
# :. dy/dx=(38x^4+38x^2 - 57x^4-16x^2+1 )/(x^3+x)^2 #
# :. dy/dx=(-19x^4+22x^2 +1 )/(x^3+x)^2 #
# :. dy/dx=-((19x^4-22x^2 -1 ))/(x^3+x)^2 #
At extrema,
This is quadratic in
To solve this we use the quadratic formula
# x=(-b+-sqrt(b^2-4ac))/(2a)#
to find two solutions for
# x=+-1.096 # (3dp)
Due to the symmetry about O, we only need to examine one of these critical points as the other will be the opposite. Let's look at
By inspection (using a calculator with
When
Hence, Critical points are:
Maximum at
Minimum at