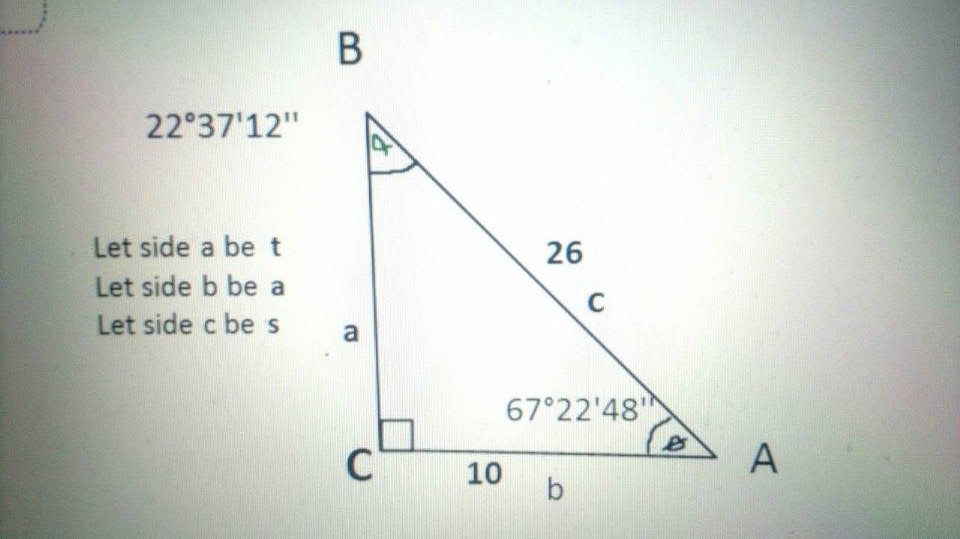
Given a right triangle #triangle ABC# with #C=90^circ#, if b=10, c=26, how do you find a?
2 Answers
Explanation:
multiply L.H.S and R.H.S. by 10
Check: using Pythagoras
Complete the ratio
to get
Explanation:
There are right-angled triangles whose sides are rational numbers.
The sides are known as "Pythagorean Triples".
If you recognise that two given sides are in one of the triples, you can simply write down the length of the third side by simple multiplying.
Some of the common triples are:
Note that the following are all in the ratio:
There are infinitely many triples which can be created.
In this case we have 2 sides as
The third side will therefore be 12 to complete the triple


