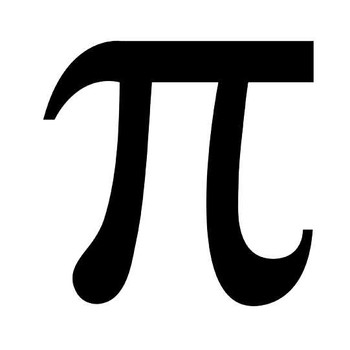
How to find solution set in #RR^3# of #x-pi=5#?

Question

Using the method of substitution, obtain the solution set in #RR^3# of the following:

1) #x-pi=5#
2) #2x-y+z=1, x-2y+z=3, y=sqrt2-z#
3) #x-y=5, x=7, 2x-3y=5#

1654 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-03-16T21:11:10

1) #(x,y,z)=(57/7, 0, 0)#
2) #(x, y, z) = ((-3-sqrt2)/4, (sqrt2-5)/4, (5+3sqrt2)/4)#
3) #(x, y, z) = (7,2,0)#

1) #x-pi=5#
#x=5+22/7 = 57/7#
Therefore, #(x,y,z)=(57/7, 0, 0)#

2) Solving using substitution method:
#2x-y+z=1# (i)
#x-2y+z=3# (ii)
#y=sqrt2-z# (iii)

Solving (i) with substitution method we get:
#x=1/2 + sqrt2/2 - z#

Putting value of x and y into (ii) we get:
#z= (5+3sqrt2)/4#

Putting value of z into (ii) we get:
#y = (sqrt2-5)/4#

Putting value of y and z into (i) we get:
#x= -((3+sqrt2)/4)#

2) Solving using substitution method:
#x-y=5# (i)
#x=7# (ii)
#2x-3y=5# (iii)

Putting the value of x into (i) we get:
#y = 2#

Therefore, #(x,y,z) = (7,2,0)#

Is there any explanation needed here to make the statement and solution more viable with the condition given (#RR^3#)?