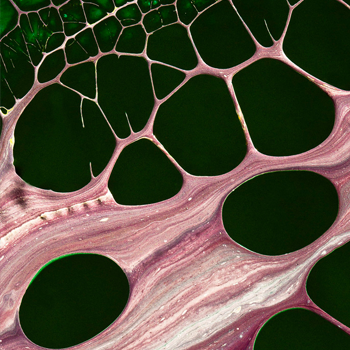
#3/x+x/(x+2)=(-2)/(x+2)#
#:.(3(x+2)+(x)(x)=-2(x))/(x(x+2))#
multiply L.H.S AND R.H.S. by #color(purple)( x(x+2)#
#:.3x+6+x^2=-2x#
#:.3x+2x+x^2=-6#
#:.5x+x^2=-6#
#:.x^2+5x+6=0#
#:.(x+3)(x+2)=0#
#:.x+3=0# or #x+2=0#
#:.color(purple)(x=-3# or #color(purple)(x=-2#
substitute #color(purple)(x=-3#
#:.3/color(purple)(-3)+color(purple)(-3)/(color(purple)(-3)+2)=(-2)/(color(purple)(-3)+2)#
#:.-1+(-3)/-1=(-2)/(-1)#
#:.-1+3=2#
#:.2=2#
substitute #color(purple)(x=-2#
#:.3/color(purple)(-2)+color(purple)(-2)/(color(purple)(-2)+2)=(-2)/(color(purple)(-2)+2)#
#:.-1.5+(-2)/0=(-2)/0#
extraneous solution, so # x # can't be #-2#