How do you divide #(x^3-8x^2+17x-10) -:(x-5)#?
2 Answers
Explanation:
N.B the quotient is the answer.
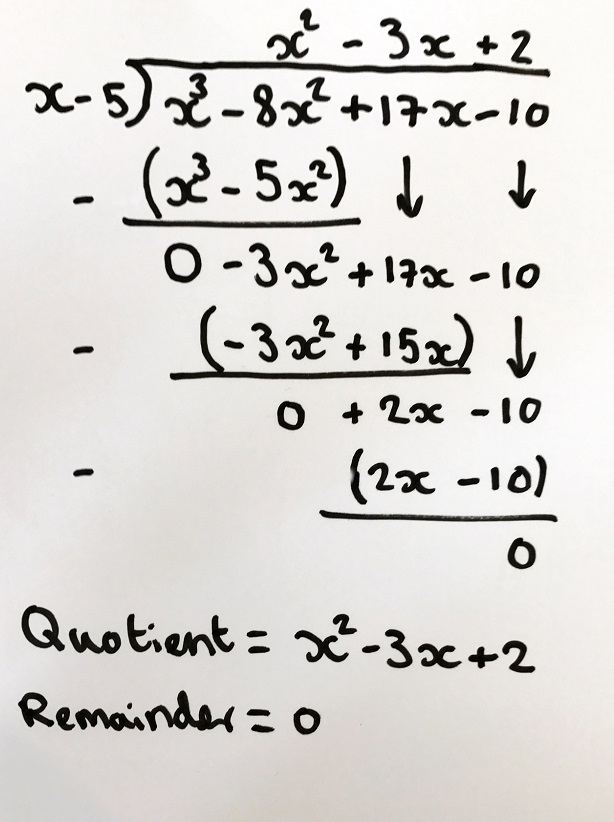
If you're unfamiliar with this technique, all you are really doing is choosing a number (can contain a variable, e.g
For this method you want to work left to right, so the first thing I want to remove is the
Explanation:
#"one way is to use the divisor as a factor in the numerator"#
#"consider the numerator"#
#color(red)(x^2)(x-5)color(magenta)(+5x^2)-8x^2+17x-10#
#=color(red)(x^2)(x-5)color(red)(-3x)(x-5)color(magenta)(-15x)+17x-10#
#=color(red)(x^2)(x-5)color(red)(-3x)(x-5)color(red)(+2)(x-5)color(magenta)(+10)-10#
#=color(red)(x^2)(x-5)color(red)(-3x)(x-5)color(red)(+2)(x-5)+0#
#rArr(x^3-8x^2+17x-10)/(x-5)#
#=(cancel((x-5))(color(red)(x^2-3x+2)))/cancel((x-5))#
#=x^2-3x+2#

