Question

A helicopter at A(6, 9, 3) moves with constant velocity. 10 minutes later, it is at B(3, 10, 2.5) Distances are in kilometres. At what angle to the horizontal does is the helicopter flying?

Answer 1

`~~8.98^o`

Explanation:

So first up we need to understand the question completely. A helicopter starts at `A(6,9,3)`, and after 10 mins, it is at `B(3,10,2.5)`. We need to find the angle between the velocity vector (which would be `vec (AB)`) and the `XY` plane.

So first we need to find the velocity vector which would be `vec (AB)`:

To find the vector between two points `M(x_1,y_1,z_1)` and `N(x_2,y_2,z_2)`, use:

`vec(MN)=((x_2-x_1),(y_2-y_1),(z_2-z_1))`

So from our points, we have:

`A(6,9,3)` and `B(3,10,2.5)`

`=>vec(AB)=((3-6),(10-9),(2.5-3))`

`=>vec(AB)=((-3),(1),(-0.5))`

This vector is the velocity vector of the helicopter in terms of km per 10 minutes (as it travels from `A` to `B` in 10 minutes)

This vector would look like:

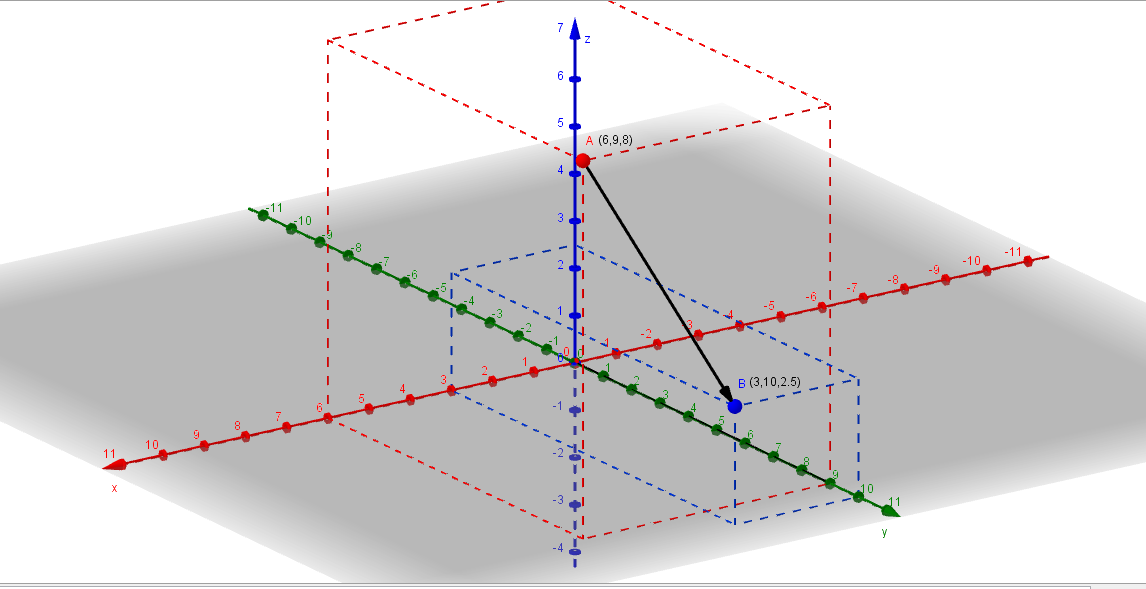
(the red dot is A and the Blue dot is B)

So we need to find the angle that `vec(AB)` makes with the XY plane.

To do this find the angle between `vec(AB)` and the vector on the XY plane which is the same as `vec(AB)`, but the `z` value is zero. Let's call this vector `a`.

`vec(AB)=((-3),(1),(-0.5))`

`=>veca=((-3),(1),(0))`

To find the angle between the two vectors `vecb` and `vecb`, use:

`costheta=(|vecb*vecc|)/(|vecb||vecc|)`

In this equation, the numerator is the modulus of the dot product and the denominator is the length of each vector.

So when we are finding the angle between `vec(AB)` and `veca`, we would use:

`costheta=(|vec(AB)*veca|)/(|vec(AB)||veca|)`

`=>costheta=(|((-3),(1),(-0.5))*((-3),(1),(0))|)/(|((-3),(1),(-0.5))||((-3),(1),(0))|)`

`=>costheta=(-3*-3+1*1+-0.5*0)/(sqrt((-3)^2+1^2+(-0.5)^2)sqrt((-3)^2+1^2+(0)^2))`

`=>costheta=(9+1+0)/(sqrt(9+1+0.25)sqrt(9+1+0))`

`=>costheta=10/(sqrt10.25sqrt10)`

`=>costheta=10/(sqrt(10.25*10)`

`=>costheta=10/sqrt102.5`

`=>theta=cos^-1(10/sqrt102.5)`

`=>theta~~8.98` (to two s.f.)