A system generated by a quadratic equation and a linear equation can have no solution, one solution, or two solutions. Explain how each possibility can occur. ?
I don't understand the two solutions part as there is many ways to explain this, can someone help me get started?
I don't understand the two solutions part as there is many ways to explain this, can someone help me get started?
3 Answers
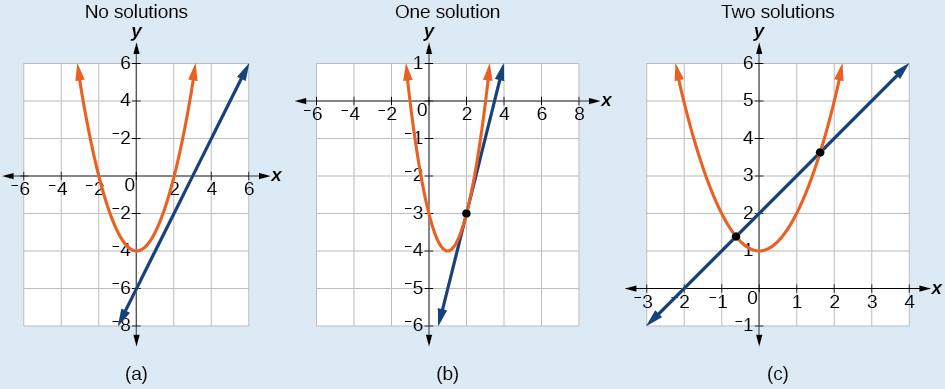
Thinking graphically, these correspond to the graph of the straight line (a) missing the graph of the parabola entirely, (b) kissing the parabola at one point or (c) cutting across the parabola and coinciding with it at 2 points.
The graphs in Fleur's answer provide a lovely illustration!
Explanation:
Graphically, a quadratic equation is a parabola and a linear equation is a straight line.
We can imagine situations where the straight line never crosses the parabola, passing above or below it (depending on the sign of the
We can imagine a situation where the straight line is a tangent to the parabola, 'kissing' it at only one point. That single point lies in both curves, and represents a single solution to the system of equations.
Finally, if the line cuts across the parabola, it will cut the parabola at 2 points (and no more than 2), and those two points will represent two solutions to the system of equations.
No solution: The line does not intersect the parabola at all.
One solution: The line intersects the parabola at one point.
Two solutions: The line intersects the parabola at two points.
This image should help!
Discriminant
Explanation:
If you are given the two equations, solve them simultaneously and then take the discriminant of the resulting quadratic.
In the form
If this is less than zero, there are no real solutions, if it equals zero, it is a repeated solution and greater than zero, 2 real solutions. Added to that, if the discriminant is a square number, the quadratic will factorise.
Sorry if formatting is poor, I'm on my phone!


