How do you find the domain and range and determine whether the relation is a function given :#x=2y^2-3#?
1 Answer
See below.
Explanation:
For y as a function of x.
Rearrange;
Add 3 to both sides:
Divide by 2:
Taking roots of both sides:
For positive root:
For the domain we must make sure the numerator is
The numerator is
So the domain is
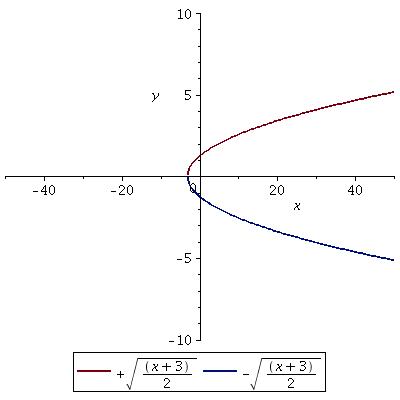
The range for the positive root is:
As
So the range is:
For negative root the domain is the same for real numbers. The range is in the reverse direction:
The determination of a function.
For positive root only a function is defined.
For negative root only a function is defined.
For
This is not a function. A function is only defined if an element in a domain is mapped onto one and one only element in a range. In the case of both roots this would map one element in the domain to two elements in the range. This is sometimes called a one to many relationship.
See graph: