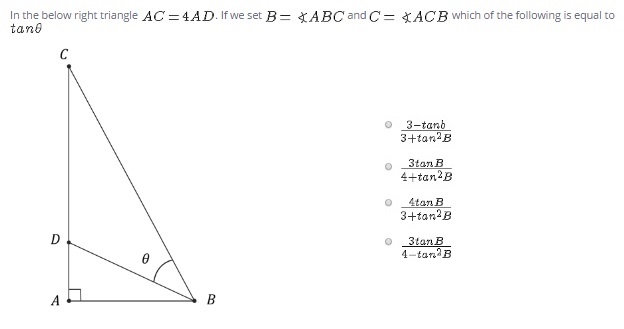
Given that #AC=4AD# or #AD=(AC)/4#
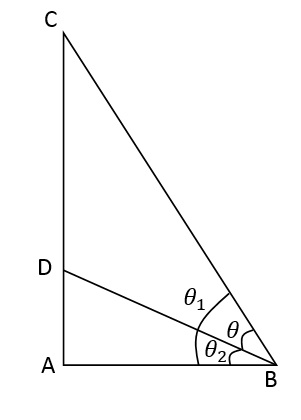
Let #angleDBC=theta_1# and #angleABD=theta_2#
Hence #theta=theta_1-theta_2#
taking #tan# both side
#tantheta=tan(theta_1-theta_2)#
#tantheta=(tantheta_1-tantheta_2)/(1+tantheta_1 tantheta_2)#
#angle ABC=B=theta_1#
in#"Triangle" DAB#
#tantheta_2=(AD)/(AB)#
replace AD by #(AC)/4#
#tantheta_2=(AC)/(4AB)#
but we know that in Triangle #CAB#
#tantheta_1=tanB=(AC)/(AB)#
So #tantheta_2=(AC)/(4AB)=1/4tanB#
Hence
#tantheta=(tanB-tantheta_2)/(1+tanB tantheta_2)#
#tantheta=(tanB-1/4tanB)/(1+tanB 1/4tanB)=((4tanB-tanB)/4)/((4+tan^2B)/4)#
4 is going to be cancel
#tantheta=(3tanB)/((4+tan^2B)#