See the diagram below.
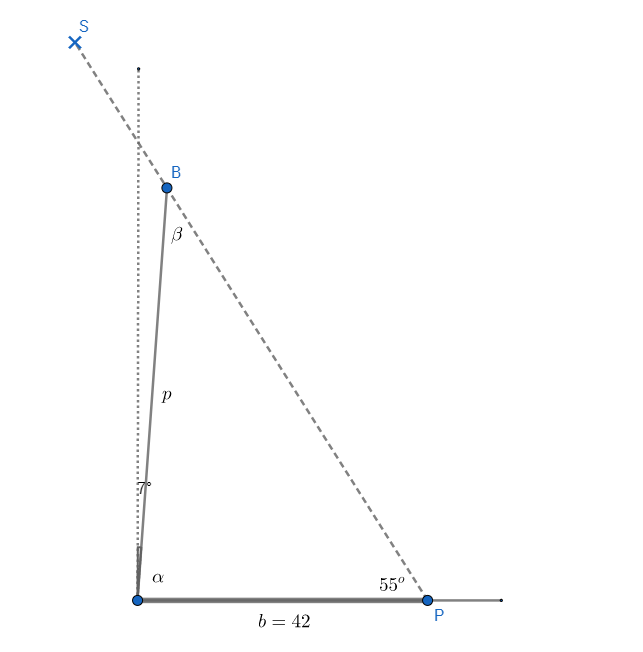
In the diagram, #alpha# represents the angle the pole makes with the ground, while point #P# marks the edge of the shadow on the ground.
From inspection, we can see that the angles #alpha#, #beta#, and #55^o# must all sum to #180^o#. Furthermore, since the pole makes an angle of #7^o# to the vertical, we know that #alpha + 7^o = 90^o#, and thus #alpha = 83^o#.
This means that we can calculate #beta#:
#alpha + beta + 55^o = 180^o #
#83^o + beta + 55^o = 180^o #
#beta = 42^o#
Since we now have #/_P#, #beta#, and #b#, and we are looking for #p#, the Law of Sines can be helpful here:
#sin P/p = sin beta/b#
#sin 55^o/p = sin 42^o/42#
#(42*sin 55^o)/(sin 42^o) = p#
#p ~~ 51.4 "ft"#

