Men have head breadths that are normally distributed with a mean of 6.0 inches and a standard deviation of 1.0 inches. If one male is randomly selected, what is the probability that his head breadth is less than 6.2 inches?
The Safeguard Helmet Company plans an initial production run of 1200 helmets. What is the probability that 100 randomly selected men have a mean head breadth less than 6.2 inches?
The Safeguard Helmet Company plans an initial production run of 1200 helmets. What is the probability that 100 randomly selected men have a mean head breadth less than 6.2 inches?
1 Answer
.5793 or 57.93%
.9772 or 97.72%
Explanation:
We want to find the probability that, in a normally distributed series, we will encounter a value that is less than the value that is .2 greater than the mean.
The z-table is great for this kind of problem.
The z-score for a head breadth of 6.2 would be .2 because the mean is 6, and the standard deviation is 1.
We can now look in our positive z-table (the one I've linked here is from chegg.com) to find the area of a normal curve to the left of
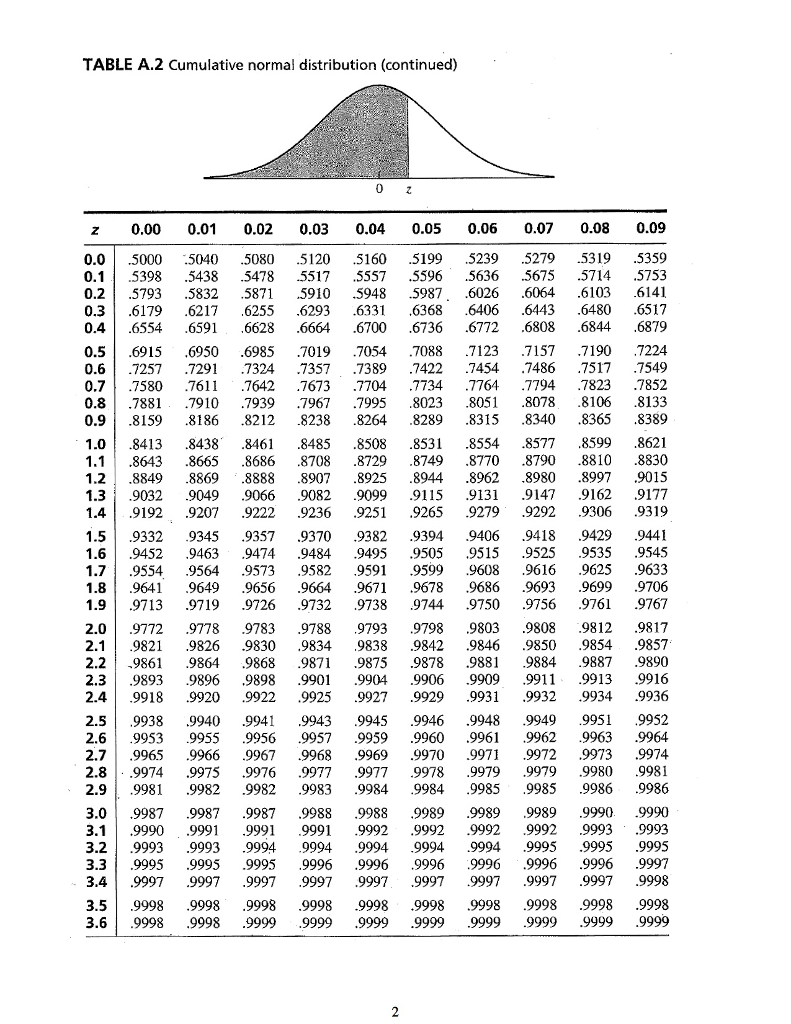
Edit: I see that this is a two-part question.
For the second part, you want to use this formula:
with
We can now use the central limit theorem.
Going back to our z table:
or a 97.72% chance.

