Question

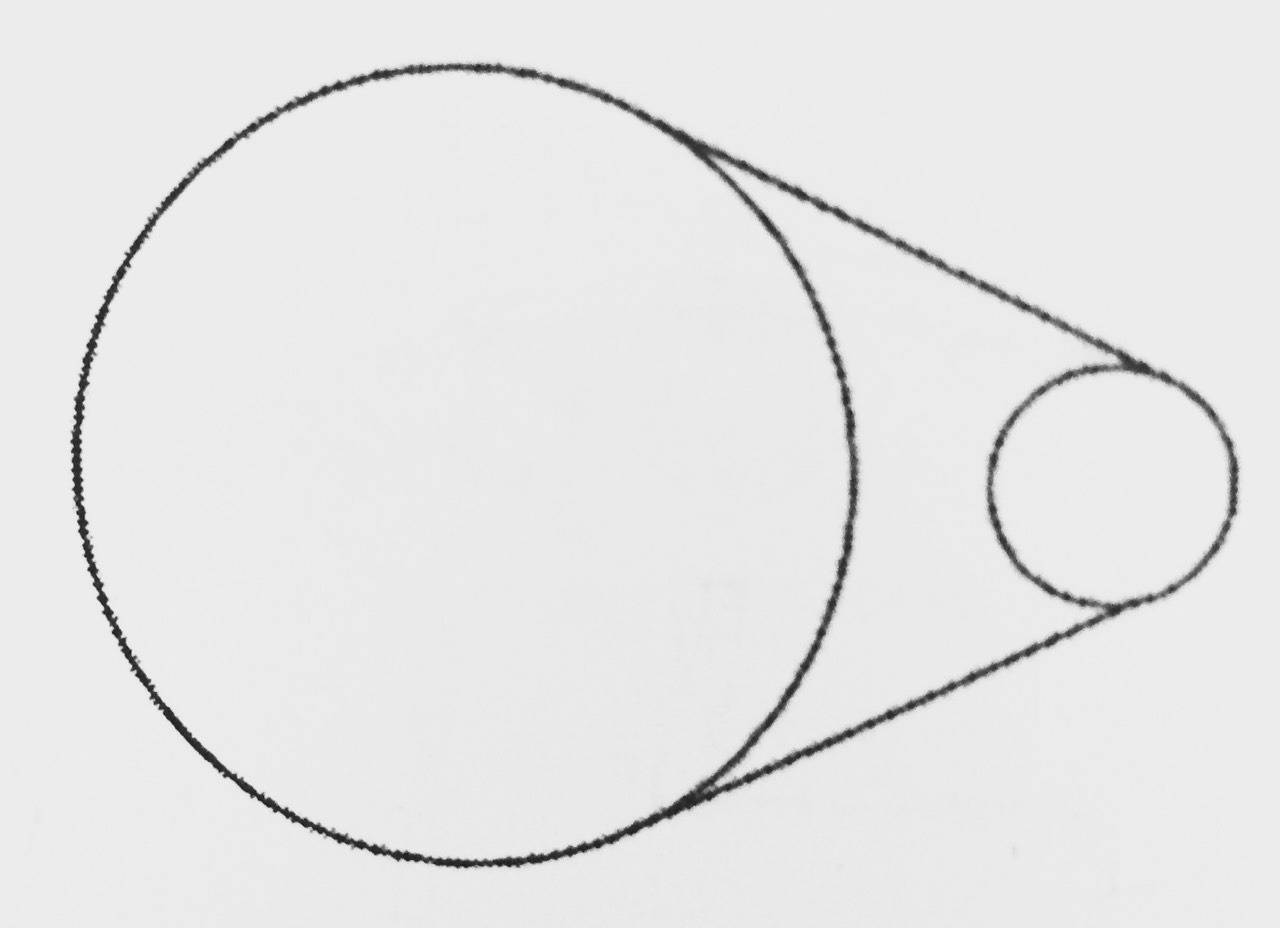
A belt is fitted tightly around two wheels of radii `20 cm` & `5 cm` which are `5 cm` apart. How do you show that the length of the belt is `30(pi + sqrt3) cm`?

Answer 1

The length of the belt is shown to be `30(pi+sqrt3)cm`.

Explanation:

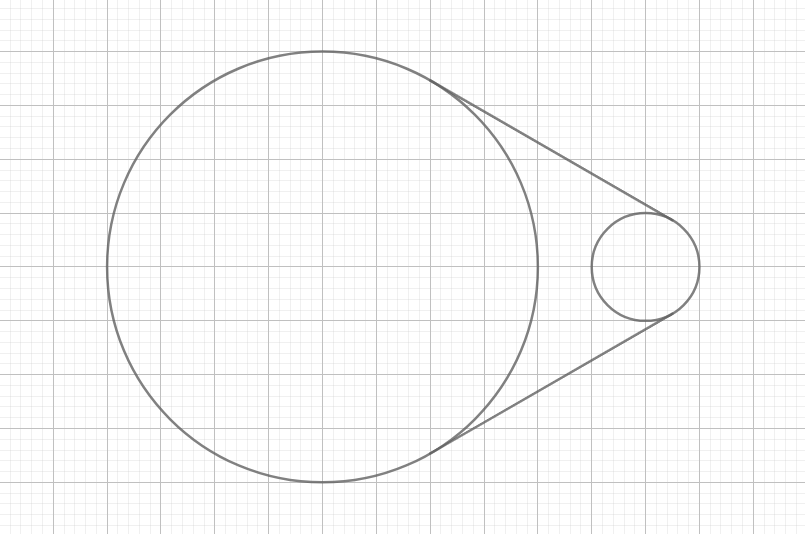
Since the belt is a tangent to the radii of both circles, we can safely say:

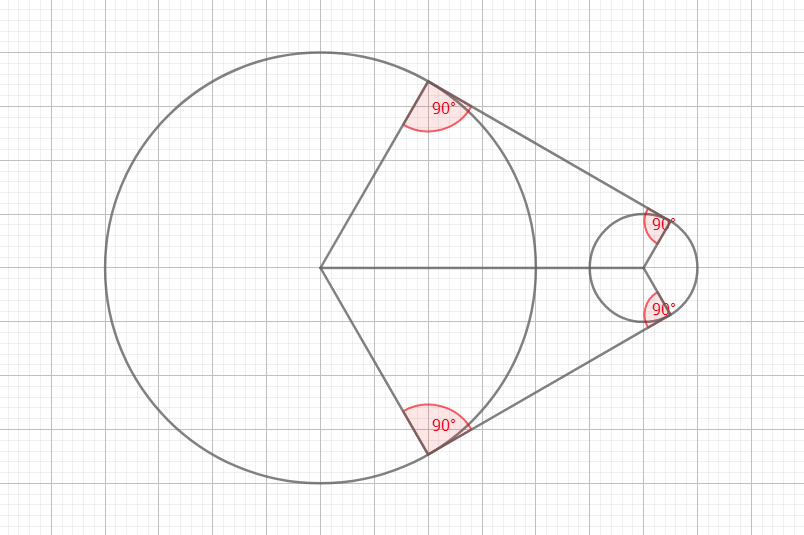
By isolating one of the trapeziums, we get this,

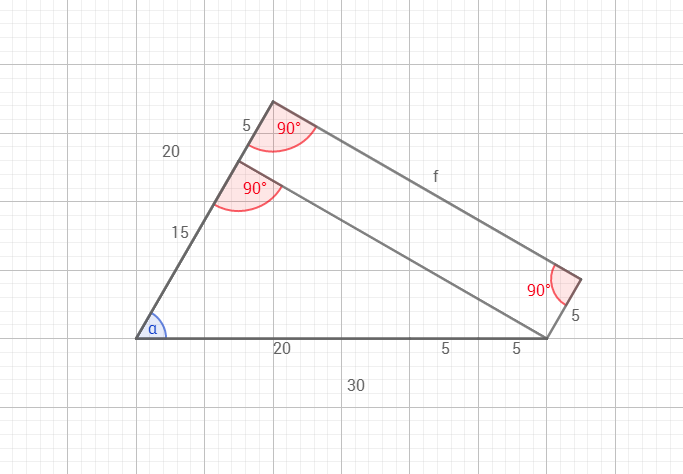
To find `f` ( a side of the belt not in contact with the circles ), use Pythagoras Theorem,

`f=sqrt(30^2-15^2)`
`color(white)(f)=sqrt675`
`color(white)(f)=15sqrt3`

To find `alpha`, use cosine,

`cos(alpha)=15/30`
`alpha=cos^(-1)(15/30)`
`color(white)(alpha)=60^@`

Therefore, we get this,

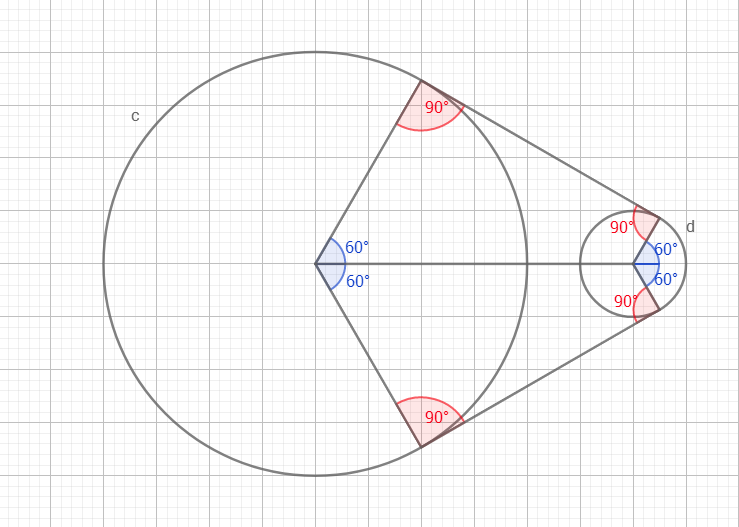
Now, let's find the `c` ( belt in contact with large circle ),

`c=(360^@-60^@-60^@)/360^@*2*pi*20`
`color(white)(c)=2/3*2*pi*20`
`color(white)(c)=80/3pi`

Now, let's find the `d` ( belt in contact with small circle ),

`d=(60^@+60^@)/360^@*2*pi*5`
`color(white)(d)=1/3*2*pi*5`
`color(white)(d)=10/3pi`

Finally, length of belt,

Length`=80/3pi+10/3pi+2*15sqrt3`
`color(white)(xxx..)=30pi+30sqrt3`
`color(white)(xxx..)=30(pi+sqrt3)`

Hence, the length of the belt is `30(pi+sqrt3)cm`, shown.

P.S. It's a tad bit long, sorry! Math is quite fun and not that pointless ( get it? here's a hint: circle ) after all!