cot765^@ = cot765∘=? sec765^@ = sec765∘=? csc765^@ = csc765∘=?
2 Answers
Explanation:
"note that "765^@=765-720^@=45^@note that 765∘=765−720∘=45∘
rArrcot765^@=1/(tan45^@)=1/1=1⇒cot765∘=1tan45∘=11=1
rArrsec765^@=1/(cos45^@)=1/(1/sqrt2)=sqrt2⇒sec765∘=1cos45∘=11√2=√2
rArrcsc765^@=1/(sin45^@)=1/(1/sqrt2)=sqrt2⇒csc765∘=1sin45∘=11√2=√2
Explanation:
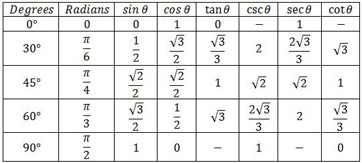
Since, they are all in the same quadrant,
With the help of this chart, we can, hence, easily solve the equation.