Student will react 50mL pipetted samples of 0.2M potassium phosphate solution with an excess of 0.12M lead II nitrate solution.?
a) which reagent is intended to be the limiting reagent?
b) what is the minimum volume of lead II nitrate solution required?
c) what volume of lead II nitrate solution should the instructor tell the students to use?
d) describe how the students can test for completeness of reaction of the limiting reagent.
Confusing! Thank you in advance :)
a) which reagent is intended to be the limiting reagent?
b) what is the minimum volume of lead II nitrate solution required?
c) what volume of lead II nitrate solution should the instructor tell the students to use?
d) describe how the students can test for completeness of reaction of the limiting reagent.
Confusing! Thank you in advance :)
1 Answer
See below.
Explanation:
a) The potassium phosphate solution is intended to be the limiting reagent because it is reacting with an excess of lead (II) nitrate solution.
A limiting reagent is the substance that will run out first in a chemical reaction, so if we have an excess (too much) amount of lead (II) nitrate that means the potassium phosphate will run out first.
b) There are multiple steps to this question.
Step 1: Write the balanced chemical reaction.
This reaction is called a double displacement reaction and it usually occurs when 2 aqeous solutions react to form a solid substance known as a precipitate. An aqeous solution is an ionic compound dissolved in water.
What determines the solubility of the products is this solubility rules table:
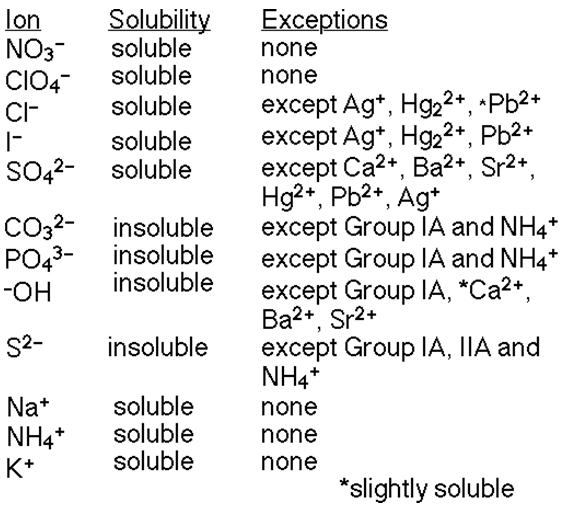
Step 2: Determine how many moles of
To do this, we apply the equation for molarity (concentration):
So for our potassium phosphate solution we can plug in the numbers from the problem:
Now, we can solve for
Step 3: Use the mole ratio between potassium phosphate and lead (II) nitrate to find how many moles of lead solution we need.
We need 3 moles of lead (II) nitrate solution for every 2 moles of potassium phosphate solution, so we have a ratio of 2:3.
We can use the inverse of this ratio to find how many moles of lead (II) nitrate we need for every 0.01 moles of potassium phosphate:
We know this is correct because
Step 4: Apply the molarity eqaution yet again, except for the numbers we have for lead (II) nitrate now.
Now we can solve for
And then divide by 0.12 on both sides:
Again, we know this is correct because it checks out when we plug it in for
c) The lead (II) solution is in excess, and we just calculated a minimum amount above. To make the solution in excess, we need more than just a minimum. Twice the minimum (
d) Weigh the amount of
The theoretical mass is the maximum amount of product that is able to be produced given a certain amount of reactant (limiting reagent).
If the actual mass is not equal to the theoretical mass, then the reaction hasn't gone to completion. It is important to note, however, that reactions won't produce theoretical mass because the real world isn't an ideal one.
If you need to show work for how to calculate the theoretical, feel free to comment and I'll be happy to help.
I hope this helps!

