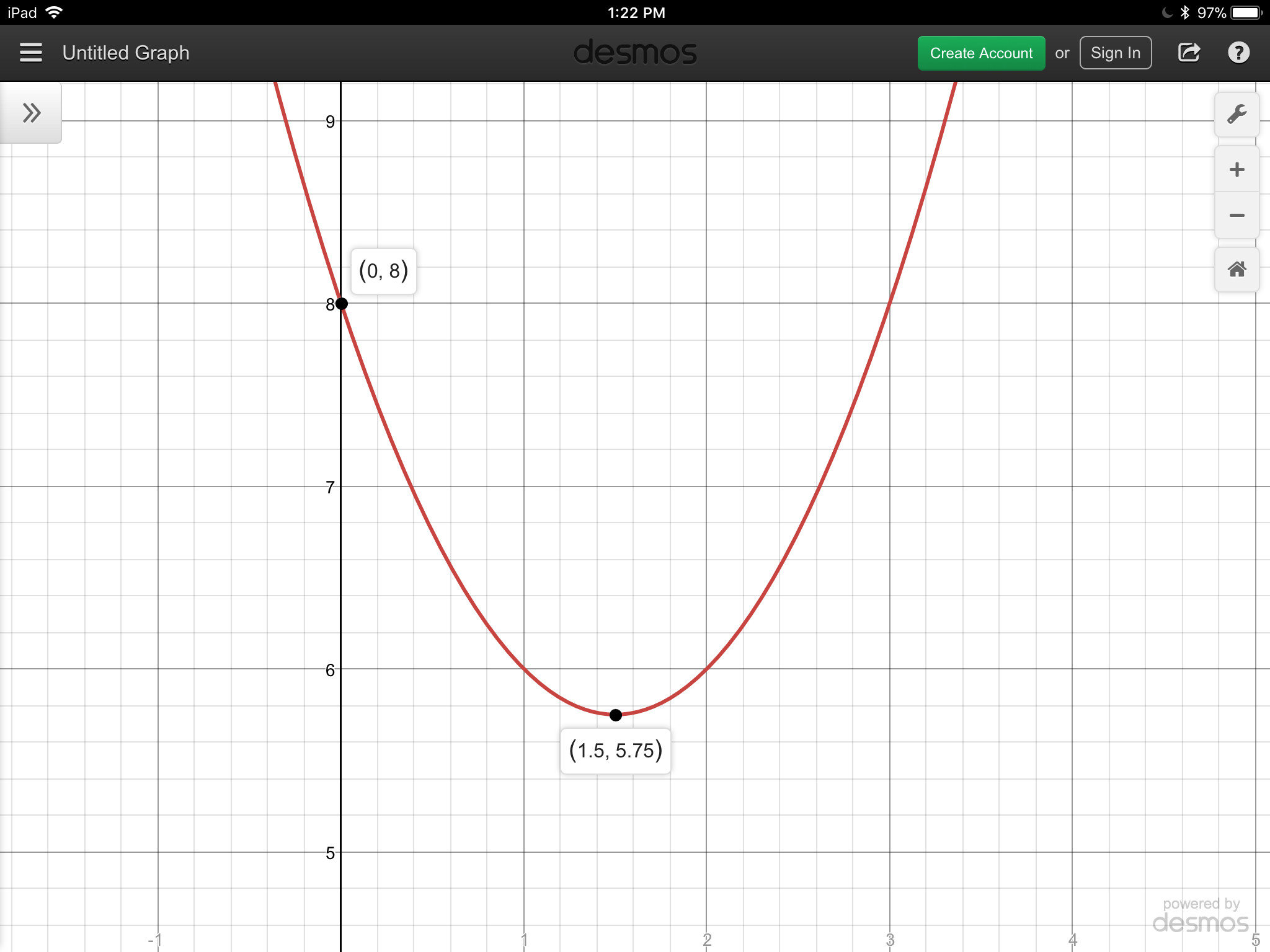
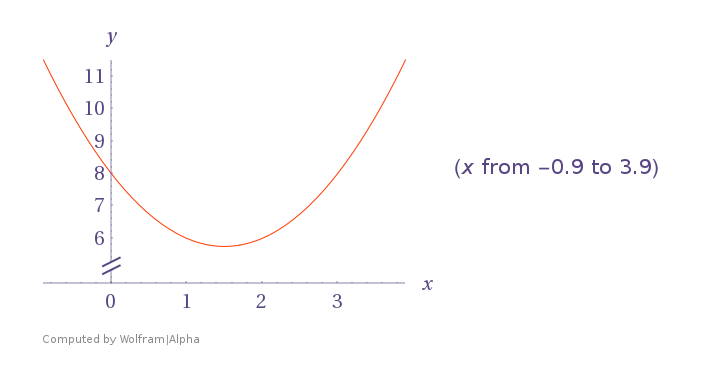
We can find the #x#-coordinate of the vertex by using the formula #-\frac{b}{2a}#, where #a# and #b# come from the standard form of #ax^2+bx+c#.
In this equation,
Plugging in yield:
#-\frac{-3}{2(1)}\quad\implies\quad \frac{3}{2}#
To find the #y#-coordinate of the vertex, plug the #x#-coordinate into the equation in standard form:
#x^2-3x+8#
#\implies (\frac{3}{2})^2-3(\frac{3}{2})+8#
#\implies \frac{9}{4}-\frac{9}{2}+8#
#\implies -\frac{9}{4}+8#
#\implies \frac{23}{4}#
#\therefore# the vertex is #(\frac{3}{2},\frac{23}{4})#.
The #y#-intercept is simply #(0,c)#, which is #(0,8)# in this case.
For a third point, we can find the axis of symmetry of the parabola, and reflect the #y#-intercept across that line.
The axis of symmetry is the vertex’s #x#-coordinate, which is #1.5#.
#\therefore# the third point will have an #x#-coordinate of #1.5\cdot 2#, which is #3#. The #y#-coordinate is the same as the #y-#-intercept, which is #8#.
#\therefore# a third point is #(3,8)#.
That’s all we need to graph a parabola.