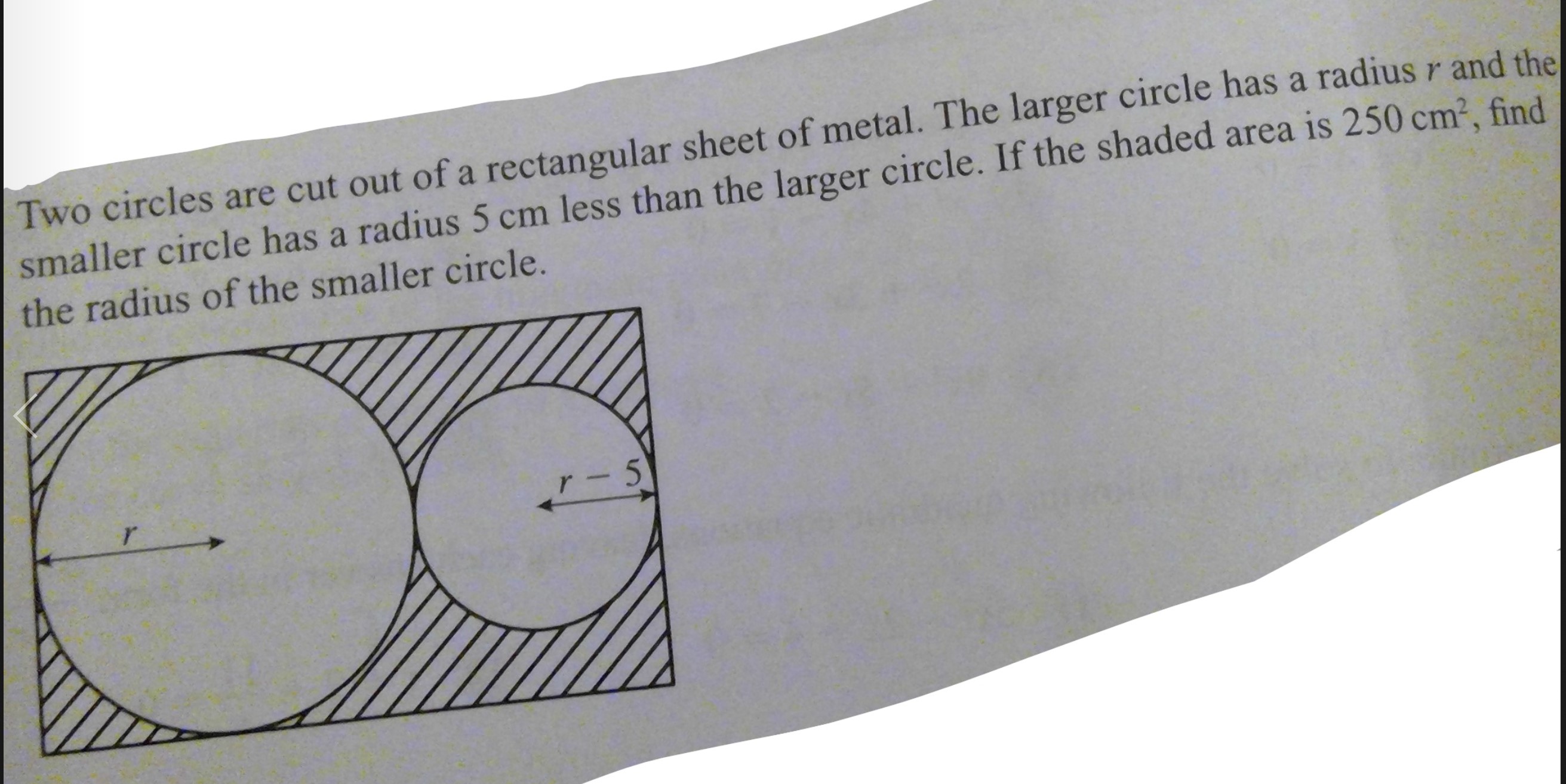
Using the radii of the circles, we can create an equation for the box using length (#l#) and width (#w#).
#l = r + r + (r - 5) + (r - 5) = 4r - 10#
#w = r + r = 2r#
As the area of a rectangle is length times width (#l*w#), we can find the total area (#A_"rectangle"#) of the rectangle in terms of r:
#A_"rectangle" = l*w #
#A_"rectangle" = (4r - 10) * 2r = 8r^2 - 20r#
The area of the shaded region is #250cm^2#, so the area of the circles (#A_"circles"#) would be the area of the rectangle minus the area of the shaded region:
#A_"circles" = A_"rectangle" - 250#
#A_"circles" = 8r^2 - 20r - 250#
Another way of expressing the area of the circles would be to just use their given radii and the formula #A_"circle" = πr^2#:
#A_"circle1" = πr^2#
#A_"circle2" = π(r-5)^2 = πr^2 - 10πr + 25π#
As #A_"circles"# is just the area of the two circles added together,
#A_"circles" = A_"circle1" + A_"circle2"#
#A_"circles" = πr^2 + (πr^2 - 10πr + 25π)#
#A_"circles" = 2πr^2 - 10πr + 25π#
Now we have two equations for #A_"circles"# in terms of r, which means we can solve for r (aka what the problem is asking for):
#A_"circles" = 8r^2 - 20r - 250#
#A_"circles" = 2πr^2 - 10πr + 25π#
#8r^2 - 20r - 250 = 2πr^2 - 10πr + 25π#
#8r^2 - 20r - 250 - 2πr^2 + 10πr - 25π = 0#
#(8-2π)r^2 +(-20+10π)r + (-250-25π) = 0#
Using the quadratic formula we can solve for r (the values in the parentheses are a, b, and c respectively):
#(-(-20+10π) +- sqrt((-20+10π)^2 - 4(8-2π)(-250-25π)))/(2(8-2π))#
We can "simplify" this to get:
#((20-10π +- sqrt(400 - 400π + 100π^2 + 8000 - 1200π - 200π^2))/(16-4π))#
#((20-10π +- sqrt(8400 - 1600π -100π^2))/(16-4π))#
As you see this is still very complicated... So I would suggest using the magic of the calculator at this point and solving for r. When you do so, you get:
#r = 10.9, - 17.6#
As r can't be negative because the problem is talking about geometry, r must equal 10.9cm.
The radius of the smaller circle is then r - 5 (as shown in the picture):
#r_"small" = r - 5 = 10.9 - 5 = 5.9#