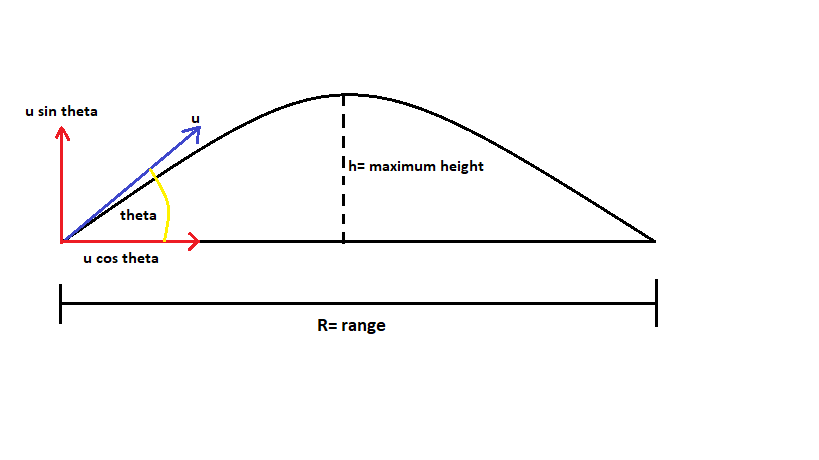
If a particle is thrown at an angle of #theta# w.r.t horizontal with a velocity # u#,it follows projectile motion(see the diagram) before landing.
the,upward motion is dure to the vertical component of velocity i.e #u sin theta# hence it goes upward,until velocity becomes zero due to the downward acceleration due to gravity,and then comes back.
and its horizontal motion is due to the #u cos theta# component.
Now,suppose it takes time #t# to reach its highest point, so using #v=u-at# we get, #t=(u sin theta)/g#,now it will take the same time for coming down,so total time of flight becomes, #T= (2 u sin theta)/g#
Now,in this time #T# if the particle horizontally goes a distance of #R# (range) ,we can say, #R= u cos theta *T # (using #s=vt# , as moving with constant velocity)
Now,putting the value of T we get,
#R= (u cos theta)(2u sin theta)/g# or,#(u^2 sin 2 theta)/g#


