Question

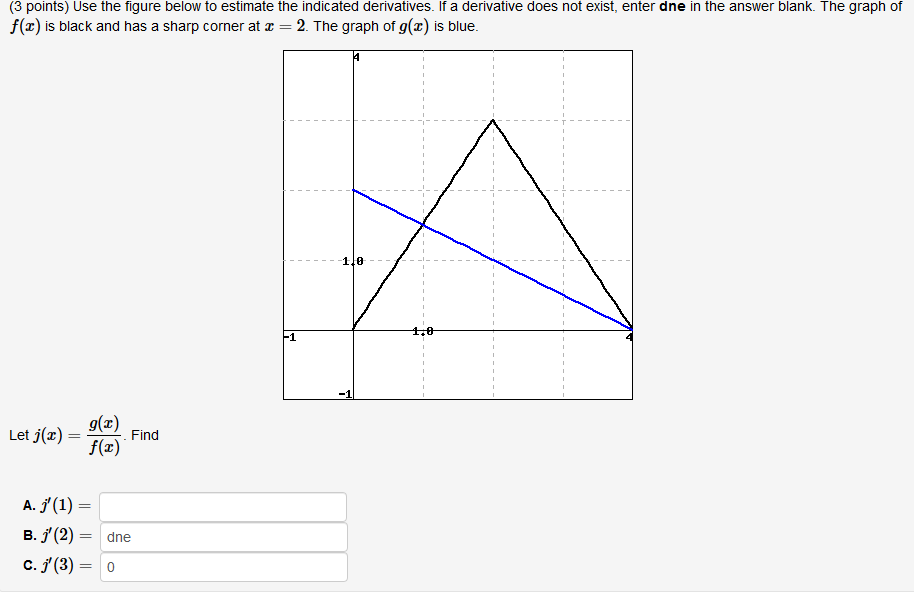
Another graph involving differentiation. This time I got j'(2) and j'(3) right, but I need help finding j'(1). Can someone help please?

Answer 1

`-4/3`

Explanation:

This question is implicitly attempting to test you on the quotient rule.

We're given that `j(x) = g(x)/f(x)`. Hence:

`j'(x) = [g'(x)*f(x) - f'(x)*g(x)]/[f(x)]^2`

This is just applying the quotient rule formula.

Now, we're being asked to find `j'(1)`. Now let's look at the graph, and list everything we know about `f(x)` and `g(x)` at `x = 1`:

`f(1) = g(1) = 3/2`
We can draw this relationship since f(x) and g(x) intersect at x = 1.

`f'(1) = 3/2`
`g'(1) = -1/2`
Note that since both f(x) and g(x) are linear functions, we can simply use the slope formula to find their derivatives.

Now, we plug all this into the formula:

#j'(1) = [g'(1)xxf(1) - f'(1)xxg(1)]/[f(1)]^2 = [(-1/2) xx (3/2) - (3/2)xx(3/2)]/[(3/2)^2]#
`= -4/3`

If you need some additional help/practice on using the quotient rule, I'd encourage you to take a look at some of my videos on the subject:

Hope that helps :)