B=20° C=75° b=5 solve the triangle?

When I set up the equation using law of sines it looked like this 5/sin(20)=x/sin(75)
then x=-2.124 what did I do wrong?

1 Answer
Anthony R.
Feb 28, 2018

#/_A=85^@#
#a~~14.56#
#c~~14.12#

Explanation:

You're calculator is in radians! That's why. It should be in degrees.

You are on the right track however!

Let's draw the triangle [Note: This is not to scale]:
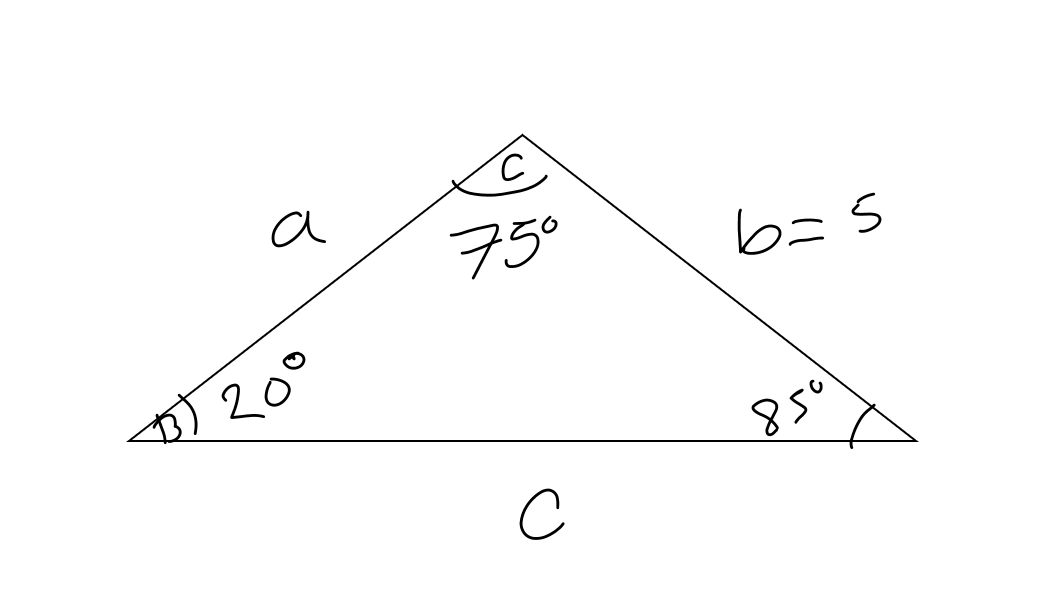
enter image source here
The missing angle can be found by knowing that the sum of the internal angles of any triangle is always equal to #180^@#

So #/_A=85^@#

The law of sines states:

#a/sinA=b/sinB=c/sinC#

We know:

#b=5#
#/_A=85^@#
#/_B=20^@#
#/_C=75^@#

What we want to know is

#a=?#
#c=?#

We can find the missing sides using the law of sines. So to find #a#

#a/sinA=b/sinB#

Pluggin in what we know:

#a/sin(85^@)=5/sin(20^@)#

#a/sin(85^@)=14.619022#

#a=sin(85^@)*14.619022#

#color(red)(a~~14.56#

Similarly for #c#:

#b/sinB=c/sinC#

#5/sin(20^@)=c/sin(75^@)#

#14.619022=c/sin(75^@)#

#sin(75^@)*14.619022=c#

#color(red)(14.12~~c#

Related questions
Impact of this question
1920 views around the world
You can reuse this answer
Creative Commons License