Let's make a sketch. We'll call the turning angle, IE, the point at which the captain must turn some degrees to go straight for island #B,# angle #C.#
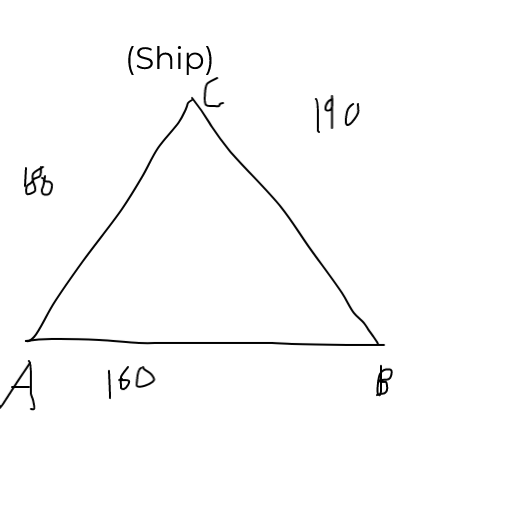
It looks like we have all sides of our triangle, but no angles. In such a case, we can use the Law of Cosines , which tells us that
#a^2=b^2+c^2-(2bc)cos(A)# where #a, b, c# are sides of the triangle and #A# is the angle opposite to side #a.#
In our case, we want angle #C,# so we can rewrite the formula like as follows:
#c^2=a^2+b^2-(2ab)cos(C)#
Side #c# is the side opposite angle #C,# which is #160.#
#a# is the side opposite angle #A,# which is #190.#
#b# is the side opposite angle #B,# which is #180.#
Plugging in, we get:
#160^2=190^2+180^2-(2*180*190)cos(C)#
Let's solve for #C:#
#160^2-190^2-180^2=-(2*190*180)cos(C)#
#-42900=68400cos(C)#
#cos(C)=-42900/68400#
#C=cos^-1(-42900/68400)approx128.84°#



