How do you find the sum of the infinite geometric series 8 + 4 + 2 + 1 +...?
1 Answer
Mar 3, 2018
Explanation:
This converging geometric progression is a special one.
Common multiple is
When you find the sum of this G.P
Basically, you need to prove
Proof:
Multiply
Subtract
As you increase the value of
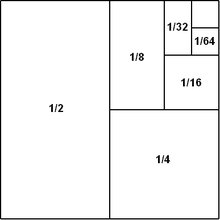
There's one more proof which is easy to understand and really amazing.
Given below is a square of side 1unit. First cut it half, then