The wavefunction is depicted as #Psi(r,t)#, where r is basically the spatial dimensions you account. For simplicity, people like to only keep it to one spatial dimension and one time dimension. The wavefunction tells you how a particle will behave. In fact, if you take the modulus of the wavefunction (please note that the wavefunction is a complex-function, as in it contains imaginary terms), and square it, you get the probability of a particle being at a certain place.
#int_a^b |Psi(x,t)|^2 dx# is the probability of finding the particle between x-coordinates a and b.
The Schrodinger Equation that it satisfies is as follows:
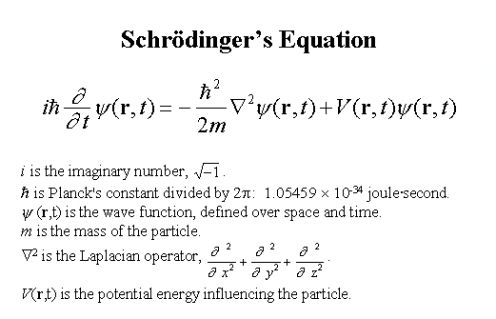
This is pretty self-explanatory. As you see, it uses differential calculus and derivatives. See the upside-down triangle? That's a differential operator, as in, it is something that differentiates a function, in this case, #Psi(r,t)#. #(dPsi)/dx# or #(dPsi)/dy# or #(dPsi)/dz# or #(dPsi)/dt# means you're measuring the rate of change of the wavefunction #Psi(r,t)# with respect to the variables x, y, z, t respectively. With respect to simply means you're measuring how they change as you move along the x, y, z, and t axes.
#V(r,t)# is the potential energy of the particle. Remember, particles like to have a low potential energy (for example, we tend to fall to lose gravitational potential energy, also because of gravity in general), so that definitely affects the behavior of the particle.
It can be noted that typically, the wavefunction has a form like:
#Ae^(i(kx-omegat))#, where A is the amplitude, or height of the wave.
#k = (2pi)/lambda#
#omega = 2pif#
#f = v/lambda#
v is the velocity of the wave, or particle, because wave-particle duality and that jazz, I think.
Also, one last thing:
#int_-oo^oo Psi(x,t) dx = 1#
This is known as normalization. Why does this make sense? Because there's a 100% chance of the particle being at any point on the interval #(-oo, oo)#.
Finally, something interesting.
#Psi(x,t) = Psi_1(x,t) + Psi_2(x,t)#
Basically, given any two wavefunctions, you can add them together to get a third wavefunction. This is known as the Principle of Superposition.


