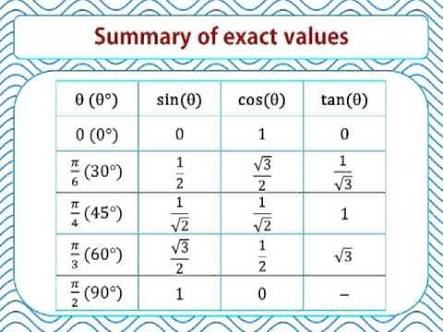
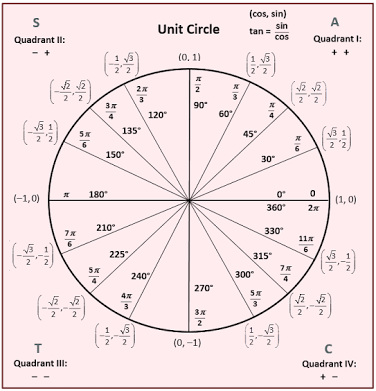
It is possible to find the exact value of #cos(pi/4)# by constructing a right triangle with one angle set to #pi/4# radians.
First, let's convert radians into degrees
#pi/4" rad"=pi/4 " rad" * 180^"o"/(pi)* "rad"^-1=45^"o"#
Now let's draw a right triangle with one of the acute angles set to #45# degrees. Remeber that these two angles would be supplementary, meaning that their sum would be #90^"o"#. As a result, the other angle in this triangle would also be #45^"o"#, making an isosceles right triangle.

By setting the length of one of the sides adjacent to the right angle to #1# and applying the Pythagorean theorem, you'll find the length of the hypotenuse #sqrt(1^2+1^2)=sqrt(2)#.
Thus #cos(pi/4)=cos(45^"o")=("adj.")/("hyp.")=1/sqrt(2)=sqrt(2)/2#.