Can the equation be solved ?
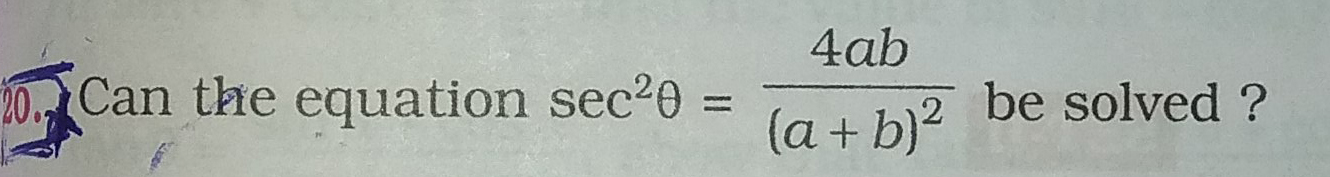
1 Answer
Apr 13, 2018
The equation does have a solution, with
Explanation:
First of all, note that
Then, consider the right-hand side. For the equation to have a solution, we must have
{since
The only solution is when
Now, substitute
Thus, the equation does have a solution, with
(If

