How do you solve #2cosx+3=0#?
2 Answers
Explanation:
Given:
#2 cos x + 3 = 0#
Subtracting
#cos x = -3/2#
This is outside the range
What about complex solutions?
Euler's formula tells us:
#e^(ix) = cos x + i sin x#
Taking conjugate, we get :-
#e^(-ix) = cos x - i sin x#
Hence on adding above two equations we get :-
#cos x = 1/2(e^(ix) + e^(-ix))#
So the given equation becomes:
#e^(ix) + e^(-ix) + 3 = 0#
Multiplying by
#0 = 4(e^(ix))^2+12(e^(ix))+4#
#color(white)(0) = (2e^(ix))^2+2(2e^(ix))(3)+9-5#
#color(white)(0) = (2e^(ix)+3)^2-(sqrt(5))^2#
#color(white)(0) = (2e^(ix)+3-sqrt(5))(2e^(ix)+3+sqrt(5))#
So:
#e^(ix) = (-3+-sqrt(5))/2#
So:
#ix = ln((-3+-sqrt(5))/2) + 2kpii#
#color(white)(ix) = +-ln((3+sqrt(5))/2) + (2k+1)pii#
for any integer
So:
#x = (2k+1)pi +- ln((3+sqrt(5))/2) i#
No solutions for
Explanation:
Subtract
This show that the equation has no real solutions. We know this because:
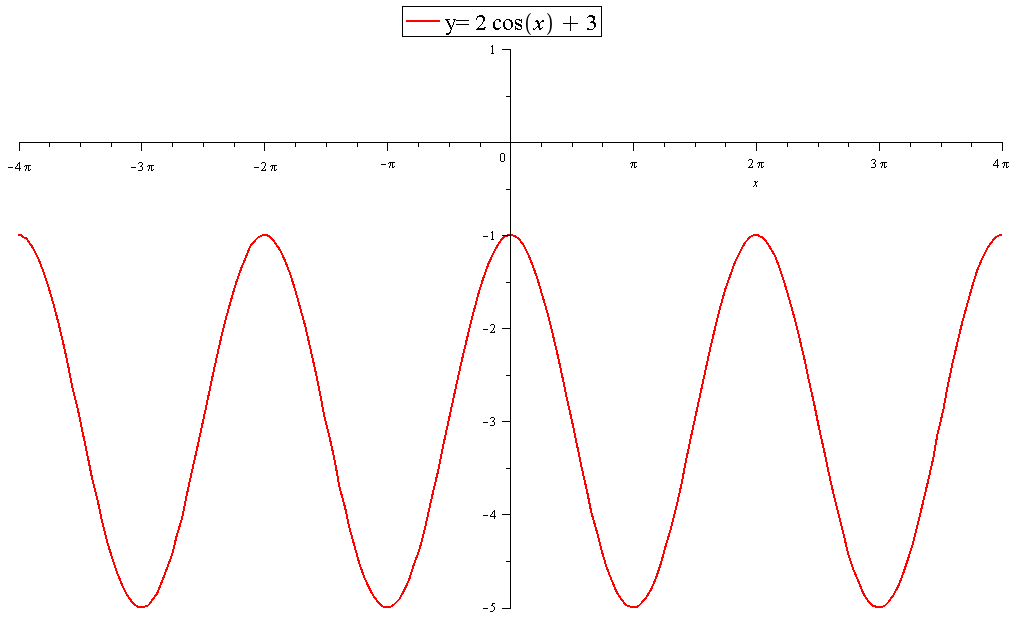
The graph of