How do you simplify #(sqrt12 - sqrt2)(sqrt12 + sqrt2)#?

Question

2684 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-04-28T17:02:54

#(sqrt12-sqrt2)(sqrt12+sqrt2)=10#

To simplify #(sqrt12-sqrt2)(sqrt12+sqrt2)#, we use the difference of two squares

#(a+b)(a-b)=a^2-b^2#

So

#(sqrt12-sqrt2)(sqrt12+sqrt2)=(sqrt12)^2-(sqrt2)^2=12-2=10#

Answer 2 · 2018-04-28T17:15:21

#10#

#(sqrt12 - sqrt2)(sqrt12 + sqrt2)#

There are two ways to solve this, and I'm going to show the longer way first.

METHOD 1:

To solve this, we distribute and expand using the FOIL method:
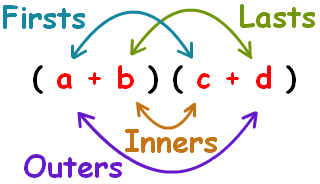
enter image source here
First, multiply the "firsts":
#sqrt12 * sqrt12 = sqrt144 = 12#

Then the "outers":
#sqrt12 * sqrt2 = sqrt24#

Then the "inners":
#-sqrt2 * sqrt12 = -sqrt24#

Finally the "lasts":
#-sqrt2 * sqrt2 = -sqrt4 = -2#

When we combine these expressions we get:
#12 + sqrt24 - sqrt24 - 2#

The #sqrt24# and #-sqrt24# cancel each other out, so we're left with:
#12 - 2#

Which simplifies down to:
#10#

METHOD 2:

To solve this, we use:
enter image source here

This expression #(sqrt12 - sqrt2)(sqrt12 + sqrt2)# is in the form #(a-b)(a+b)#, which is the same as #(a+b)(a-b)#.

As we can see, this is equivalent to #a^2 - b^2#, so it becomes:
#sqrt12^2 - sqrt2^2#

Simplify:
#12 - 2#

So the final answer is:
#10#

Hope this helps!