What is #int ln4x^2dx#?

Question

3444 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-05-05T14:01:23

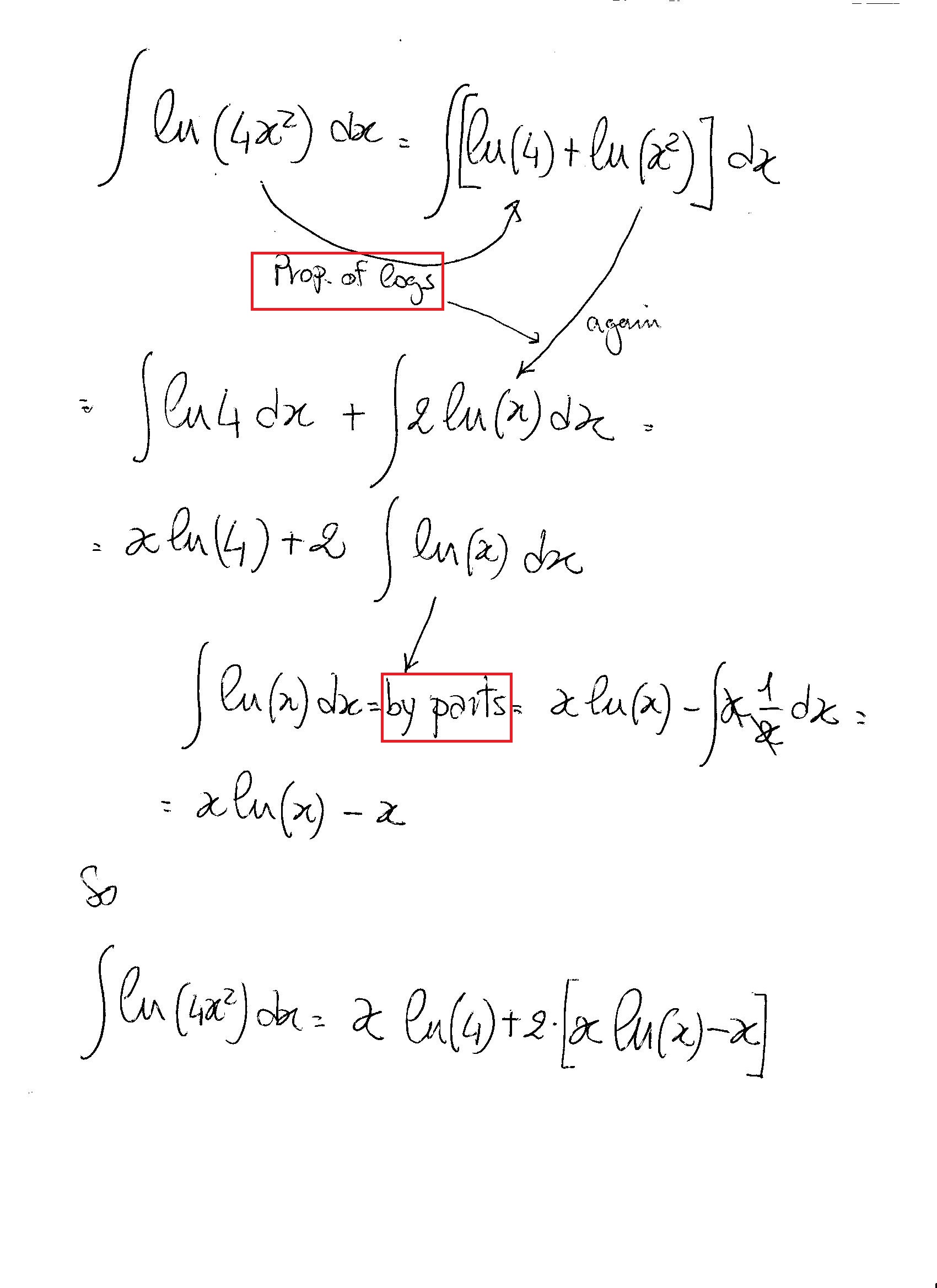
I tried this:

I used some properties of logs first to manipulate the integrand:
enter image source here

Answer 2 · 2018-05-05T14:05:45

#int ln(4x^2)\ dx = x [ ln ( 4 x^2 ) - 2 ] + C#

The key to solving this integral is to know that

#ln(4x^2) = ln 4 + ln x^2 = ln 4 + 2 ln x#.

With this, we get

#int ln(4x^2)\ dx =#
#= ln 4 int dx + 2 int ln x\ dx =#
#= x ln 4 + 2 int ln x\ dx#.

Next, we view the remaining integrand as #1 \cdot ln x#, and use integration by parts with #u' = 1# and #v = ln x#. With this, we get

#x ln 4 + 2 [ x ln x - int dx ] =#

#= x ln 4 + 2 x ln x - 2x + C =#

#= x [ ln ( 4 x^2 ) - 2 ] + C#.