Question

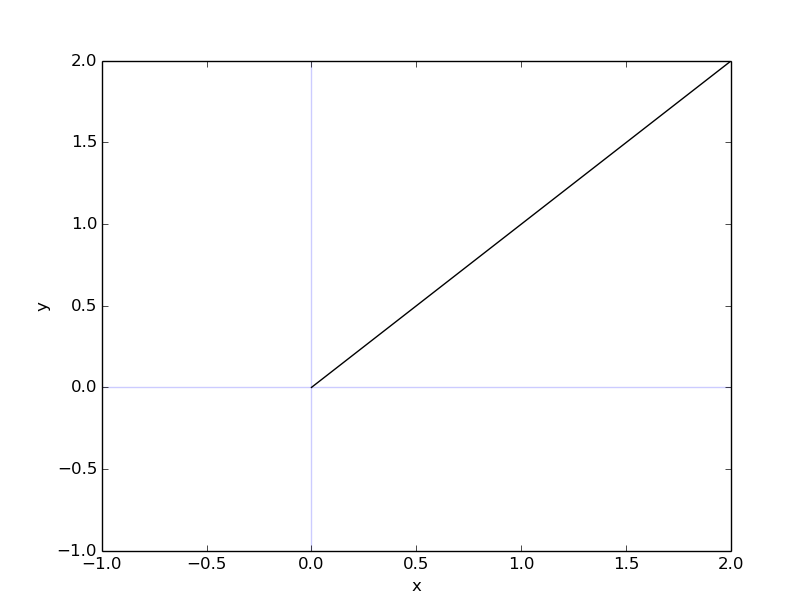
How do you graph `y=e^(ln x)`?

Answer 1

`y=e^(ln(x))`

Explanation:

The natural logarithm function, if considered as a real-valued function of a real variable, is the inverse function of the exponential function, leading to the identities:
`e^{\ln x}=x\qquad \text{if } x>0 \qquad e^{\ln x}=x\qquad \text{if }x>0`
`\ln(e^{x})=x`
Like all logarithms, the natural logarithm maps multiplication into addition:
`\ln(xy)=\ln x+\ln y`

from natural logarithm.