Question

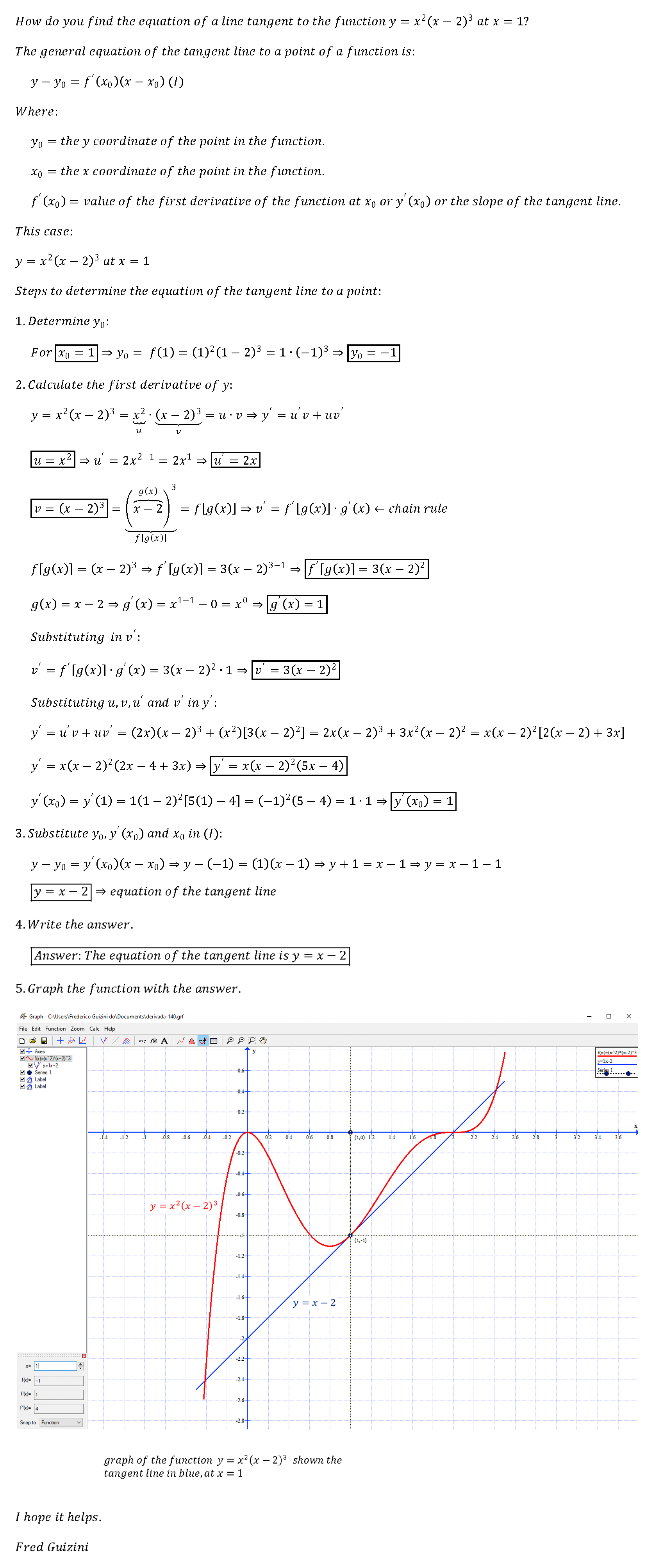
How do you find the equation of a line tangent to the function `y=x^2(x-2)^3` at x=1?

Answer 1

The equation is `y=9x-10`.

Explanation:

To find the equation of a line, you need three pieces: the slope, an `x` value of a point, and a `y` value.

The first step is to find the derivative. This will give us important information about the slope of the tangent. We will use the chain rule to find the derivative.

`y=x^2(x-2)^3`
`y=3x^2(x-2)^2(1)`
`y=3x^2(x-2)^2`

The derivative tells us the points what the slope of the original function looks like. We want to know the slope at this particular point, `x=1`. Therefore, we simply plug this value into the derivative equation.

`y=3(1)^2(1-2)^2`
`y=9(1)`
`y=9`

Now, we have a slope and an `x` value. To determine the other value, we plug `x` into the original function and solve for `y`.

`y=1^2(1-2)^3`
`y=1(-1)`
`y=-1`

Therefore, our slope is `9` and our point is `(1,-1)`. We can use the formula for the equation of a line to get our answer.

`y=mx+b`

`m` is the slope and `b` is the vertical intercept. We can plug in the values we know and solve for the one we don't.

`-1=9(1)+b`
`-1=9+b`
`-10=b`

Finally, we can construct the equation of the tangent.

`y=9x-10`

Answer 2

I have solved this way! Please, see the answer below: