Question

Find the volume of the solid generated by revolving the region bounded by x=Siny,x=1,and x-axis about y-axis?

Answer 1

`color(purple)[V=piint_(0)^(pi/2)(1)^2-(sin^2y)dy=(pi)^2/4]`

Explanation:

show that the shaded region in the sketch below revolving about y-axis.

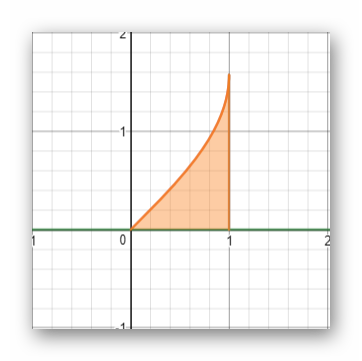
when x=1

`x=siny rArr siny=1 rArr y=pi/2`

the interval of the integral become `y in [0,pi/2]`

now set up the integral

`color(blue)[V=piint_(0)^(pi/2)(1)^2-(siny)^2*dy=piint_(0)^(pi/2)1-sin^2y*dy]`

`color(blue)[=pi[y-(y-sin(2*y)/2)/2]_0^(pi/2)=pi[(sin(2*y)+2*y)/4]_0^(pi/2)=(pi)^2/4]`