How do you solve $(11x - 5) ^ { 2} - ( 10x - 1) ^ { 2} - ( 3x - 20) ( 7x + 10) = 124$ ?

Question

4531 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-06-12T02:02:24

$color(crimson)(x = 40/53$

Follow the PEMDAS Rule to solve.

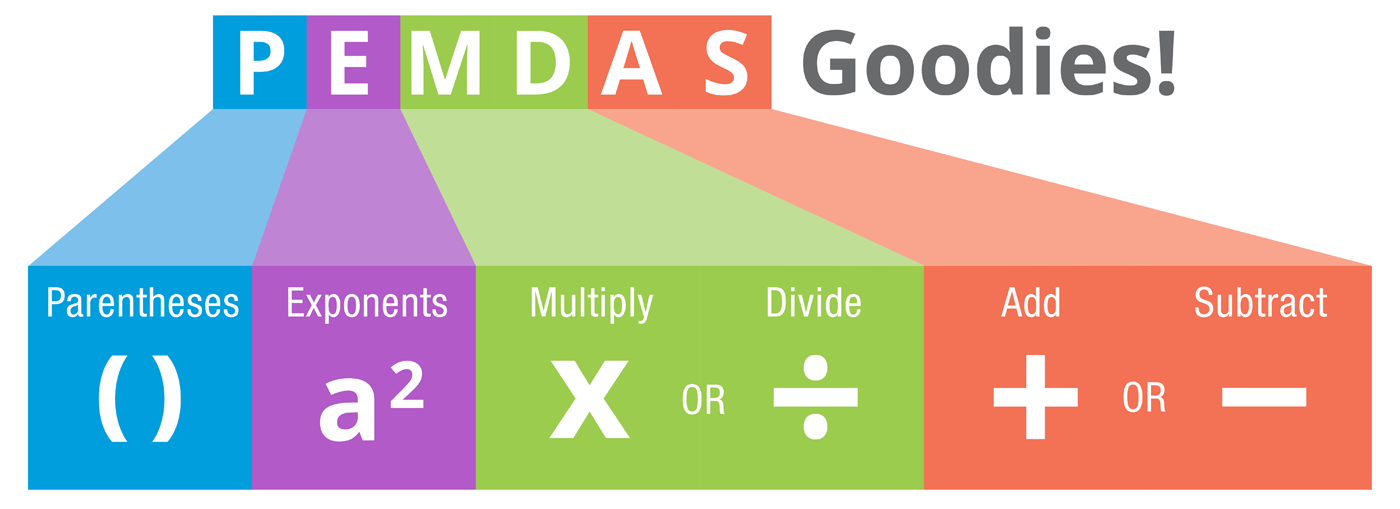
![https://www.thecalculatorsite.com/articles/units/http://how-does-pemdas-work.php](https://useruploads.socratic.org/aVZkVtW6T8yQ71ldZppO_pemdas.png)

$(11x - 5)^2 - (10x-1)^2 - (3x-2)(7x+10) = 124$

$121x^2 - 110x + 25 - 100x^2 + 20x - 1 - 21x^2 - 30x + 14x + 20 = 124, color(purple)(" Removing Braces"$

$121x^2 - 100x^2 - 21x^2 - 110x + 20x - 30x + 14x + 25 - 1 + 20 = 124, color(purple)("Bringing like terms together"$

$0 x^2 - 106x + 44 = 124, color(purple)("Adding / Subtracting"$

$106x = - 124 +44$

$106x = - 80$

$color(crimson)(x = 80/106 " or " x = 40/53, color(purple)("Simplifying"$

Answer 2 · 2018-06-12T02:08:19

$x=-5$

We have

$(11x - 5) ^ { 2} - ( 10x - 1) ^ { 2}$
$= (11x-5-10x+1)(11x-5+10x-1)$
$= (x-4)(21x-6) = 21x^2-90x+24$

and

$( 3x - 20) ( 7x + 10) = 21 x^2-110x-200$

Thus, the equation is

$(21x^2-90x+24)-(21 x^2-110x-200)=124 implies$

$20x+ 100 = 0 implies 20(x+5) = 0 implies$

$x = -5$

How do you solve (11x - 5) ^ { 2} - ( 10x - 1) ^ { 2} - ( 3x - 20) ( 7x + 10) = 124(11x - 5) ^ { 2} - ( 10x - 1) ^ { 2} - ( 3x - 20) ( 7x + 10) = 124?