For this type of problem, it's probably easiest to draw a table like this:
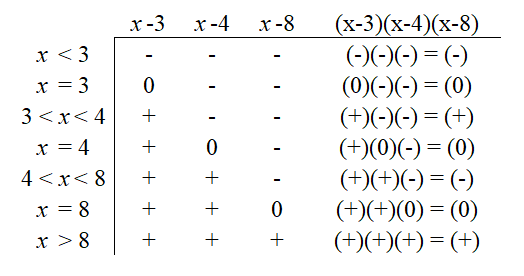
Our expression is composed of three factors, #(x-3)#, #(x-4)#, and #(x-8)#. These three factors together with their product serve as the top row heading for the table. The most left-hand column shows different possibilities for #x#, The next column to the right of this shows whether the value of #x-3# will be negative (-), zero (0), or positive (+) and the last column shows us if the product of the three factors is negative, zero, or positive.
Reading the first row below the heading we can see that when #x# is less than 3, #x-3# is negative, #x-4# is negative, and #x-8# is negative, so the product of these three factors is also negative, since a negative times a negative times a negative is negative.
Looking at the table, we can see when the product of the factors is less than or equal to zero. This is when
#x<=3#, and #4<=x<=8#.

