Question

How do you evaluate the expression `cot(-180)`?

Answer 1

`-oo`

Explanation:

remember this
for any expression like `sintheta` or `costheta` if theta exceeds `90^o` then

• step 1

resolve `theta` as `(90*n+x)` for some integer `n`

now see where `theta` lies `i.e` the quadrant number(it can also be found out by the value `n`)

quadrant 1 if `0<=theta<90` (in this quadrant all trigonometric ratios are +ve)

quadrant 2 if `90<=theta<180` (in this quadrant only `sin` and `cosec` are +ve)

quadrant 3 if `180<=theta<270` (in this quadrant only `tan` and `cot` are +ve)

  quadrant 4 if #270<=theta<360# (in this quadrant #cos# and #sec# are +ve)

you can remember it by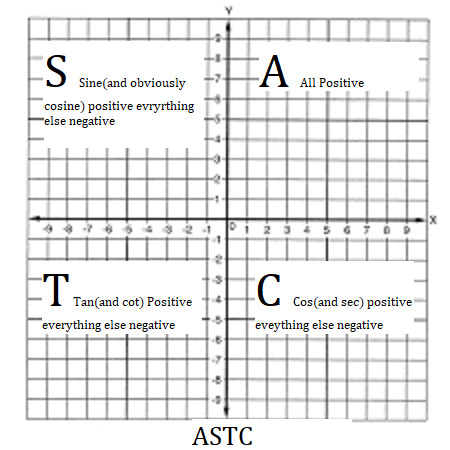
Acronym ASTC: "After School To College/Coffee/Cinema"

• step 2

Now assign the sign to the expresssion as above

• step 3

Now the key point: if `n` is odd change `sin` to `cos` and `cos` to `sin` if `n` is even do not change the ratio

you will be now left with +ve or -ve sign from step 2 a `sin` or a `cos` from step 3 and an `x` from step 1
write them in order to get the answer

coming to your question

`cot(-180)=-cot(180)=-cos(180)/sin(180)=` because #cot(-theta) = -cottheta#

`cos(180)=cos(90*2+0)`
`180^o` is in third quadrant and cos is negative there ,also 2 is even so cos will remain cos, and `x` comes out to be 0
so `cos(180)=cos(90*2+0)="- cos (0)"=-1`
similarly `sin(180)= sin(90*2+0)="- sin (0)"=0`

`cot(-180)=-cot(180)=-(-cos(0))/(-sin(0))=-1/0=-oo`