Let g(x)=#int_0^xf(t)dt# where #f# is the function whose graph is shown. Evaluate g(0), g(2), g(4), g(6) and g(12)?
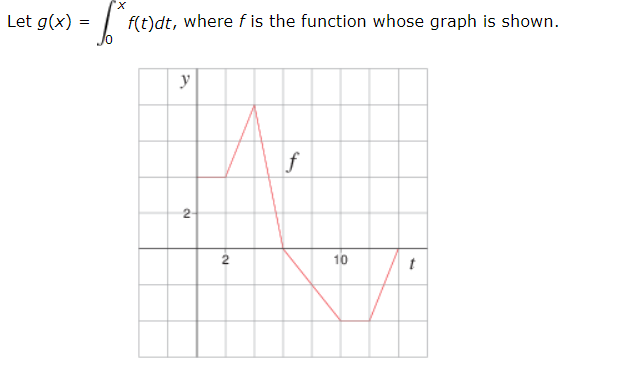
I find that g(0)=0. I think that g(2) should be 4 since the area is 4 squares but its not correct?
I find that g(0)=0. I think that g(2) should be 4 since the area is 4 squares but its not correct?
1 Answer
# g(0) = 0 #
# g(2) = 8 #
# g(4) = 20 #
# g(6) = 28 #
# g(12) = 8 #
Explanation:
We have:
# g(x) =int_0^x \ f(t) \ dt #
So that
Part (1):
# g(0) = int_0^0 \ f(t) \ dt #
# \ \ \ \ \ \ \= 0 \ \ \ # (By definition)
Part (2):
# g(2) = int_0^2 \ f(t) \ dt #
# \ \ \ \ \ \ \= 4 xx 2 \ \ \ # (Area of rectangle)
# \ \ \ \ \ \ \= 8 #
Part (3):
# g(4) = int_0^4 \ f(t) \ dt #
# \ \ \ \ \ \ \= g(2) + 1/2(4+8)(2) \ \ \ # ( + trapezium)
# \ \ \ \ \ \ \= 8 +12 #
# \ \ \ \ \ \ \= 20 #
Part (4):
# g(6) = int_0^6 \ f(t) \ dt #
# \ \ \ \ \ \ \= g(4) + 1/2(2)(8) \ \ \ # ( +#triangle# )
# \ \ \ \ \ \ \= 20+8 #
# \ \ \ \ \ \ \= 28 #
Part (5):
# g(12) = int_0^12 \ f(t) \ dt #
# \ \ \ \ \ \ \= g(6) - 1/2(8+2)(4) \ \ \ # ( + trapezium below)
# \ \ \ \ \ \ \= 28 - (10(2) #
# \ \ \ \ \ \ \= 8 #

