The sine function is periodic with period #360^@#, hence
#sin(360^@-x) =sin(-x)=-sinx#
About the tangent function; let #y=90^@ - x => x+y=90^@#.
#x# and #y# are complementary angles. We usually meet them in right triangles, where trigonometric functions are usually more familiar. As such, let #x# and #y# be the acute angles of a right triangle with sides #cc a, ccb, cc c#.
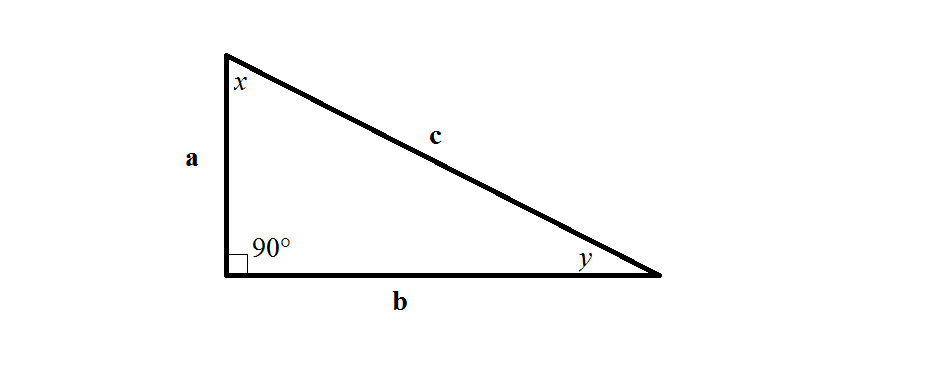
#tanx="opposite"_x/"adjacent"_x=ccb/cca#
#cotx="adjacent"_x/"opposite"_x=cca/ccb#
#tany=tan(90^@-x)="opposite"_y/"adjacent"_y = cca/ccb=cotx#
#:. tan(90^@-x)=cotx#
Hence,
#sin(360^@-x)xxtan(90^@-x)=-sinx xx cotx=-sinx xx cosx/sinx=-cosx#


