We start with the balanced chemical equation for the equilibrium.
#"CO" + "H"_2"O" ⇌ "CO"_2 +"H"_2#
Then we write the #K_P# expression.
#K_P = (P_("CO"_2)P_("H"_2))/(P_("CO")P_("H"_2"O"))#
Next, we set up an ICE table:
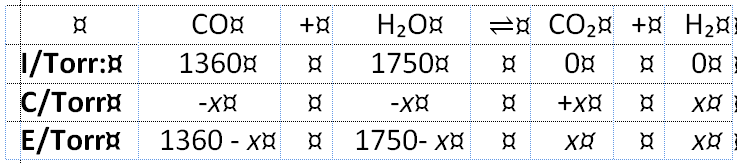
Insert these values into the #K_P# expression:
#K_P = (P_("CO"_2)P_("H"_2))/(P_("CO")P_("H"_2"O")) = (x × x)/((1360-x)(1750-x)) = 0.0611#
#x^2/(2 380 000 – 3110x + x^2) = 0.0611#
#x^2 = 145 418 – 190.02x + 0.0611x^2#
Putting all terms on the left, we get
#x^2 - 0.0611x^2 + 190.02x -145 418 = 0#
#x^2(1-0.0611) + 190.02x -145 418 = 0#
#0.9389x^2 + 190.02x -145 418 = 0#
Use your calculator or an on-line quadratic equation solver such as the one at
http://www.math.com/students/calculators/source/quadratic.htm
#x = 305#
#P_("CO") = (1360 – x)" Torr" = (1360 - 305)" Torr" = "1055 Torr"#
#P_("H"_2"O") = (1750 – x)" Torr" = (1750 – 305)" Torr" = "1445 Torr"#
#P_(CO_2) = P_(H_2) = x" Torr" = "305 Torr"#
Check: #(305 × 305)/(1055 × 1445) = 0.0610# (Close enough!)
The video below shows how to use an ICE table to solve a #K_p# problem.


