Question #edf16
1 Answer
Answer : Mechanical Energy can be transferred from/into a body through the application of a force and making it do work. Force is said to do work if the body gets displaced when the force is applied.
Work-Energy Theorem : The work-energy theorem relates the net work done by all the forces on an object to its change in kinetic energy . The net work done by all the forces is equal to the change in the object's kinetic energy.
- If
#W_{"net"} > 0# , then#K_{f} > K_{i}#
The kinetic energy of the object increases. So energy is transferred into the object. - If
#W_{"net"} = 0# , then#K_{f} = K_{i}#
The kinetic energy of the object does not change. So there is no energy transfer. - If
#W_{"net"} < 0# , then#K_{f} < K_{i}#
The kinetic energy of the object decreases. So energy is transferred out of the object.
Work Done by a Force :
When a force is applied to an object and the object gets displaced, work is said to be done by the force. It is important to understand that the direction of displacement need not be the same as the direction of force. For example, when an object moving upward and is under the influence of gravitational force, the displacement vector points up while the force vector points downward.
If
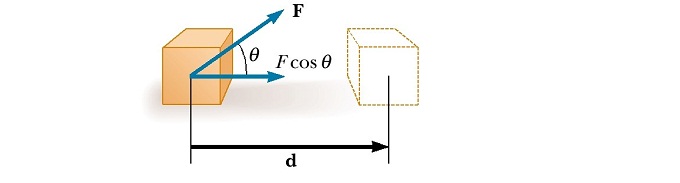
The total work done in displacing the object over a straight line distance
The work done is a signed quantity. Its sign is dependent on the angle (
- Work done is positive if
#\theta < 90^{\circ}# - Work done is zero if
#\theta = 90^{\circ}# - Work done is negative if
#\theta > 90^{\circ}#

